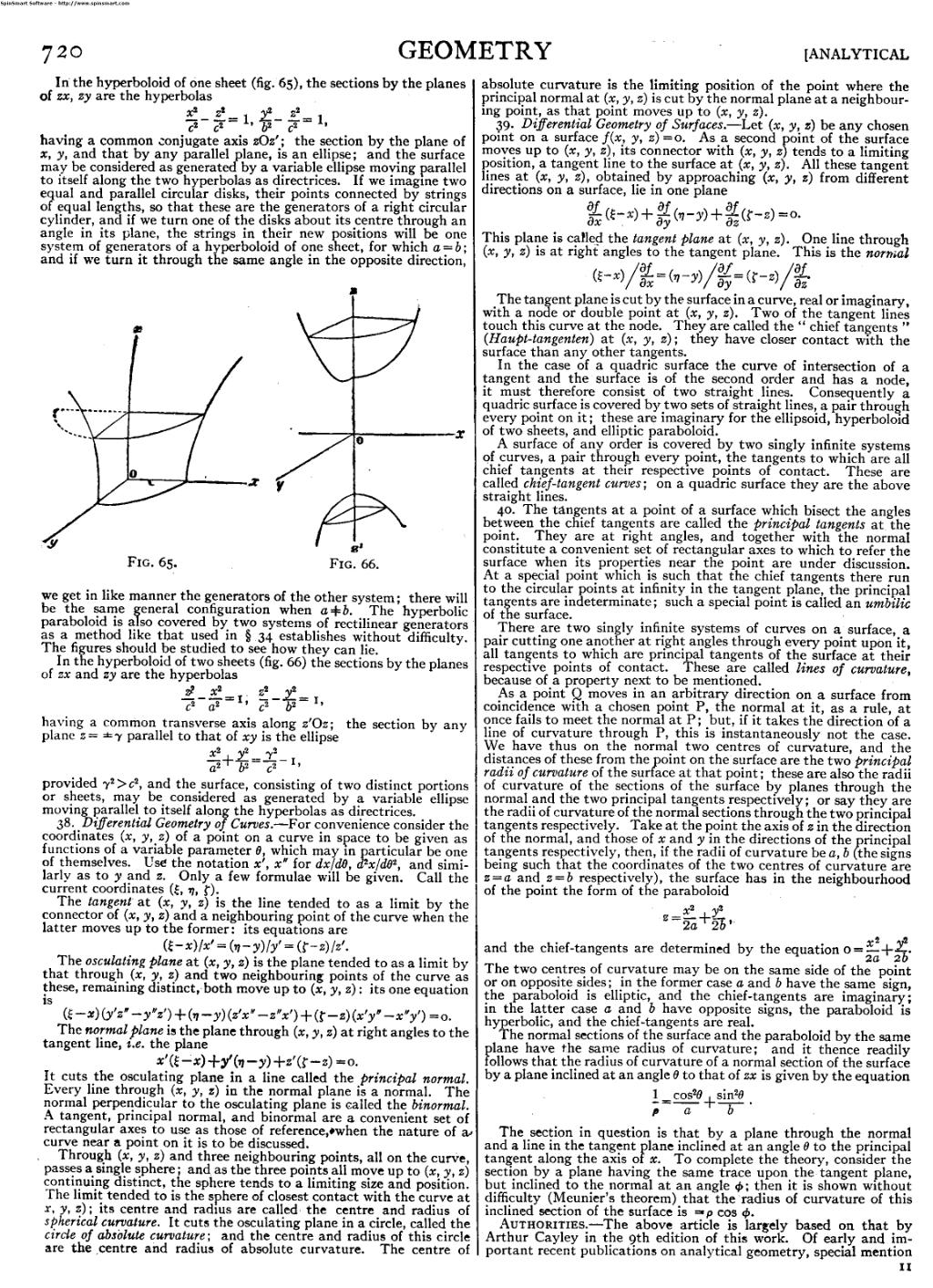
In the hyperboloid of one sheet (fig. 65), the sections by the planes of zx, zy are the hyperbolas
| x2 | − | z2 | = 1, | y2 | − | z2 | = 1, |
| c2 | c2 | b2 | c2 |
having a common conjugate axis zOz′; the section by the plane of x, y, and that by any parallel plane, is an ellipse; and the surface may be considered as generated by a variable ellipse moving parallel to itself along the two hyperbolas as directrices. If we imagine two equal and parallel circular disks, their points connected by strings of equal lengths, so that these are the generators of a right circular cylinder, and if we turn one of the disks about its centre through an angle in its plane, the strings in their new positions will be one system of generators of a hyperboloid of one sheet, for which a = b; and if we turn it through the same angle in the opposite direction, we get in like manner the generators of the other system; there will be the same general configuration when a ≠ b. The hyperbolic paraboloid is also covered by two systems of rectilinear generators as a method like that used in § 34 establishes without difficulty. The figures should be studied to see how they can lie.
 | |
| Fig. 65. | Fig. 66. |
In the hyperboloid of two sheets (fig. 66) the sections by the planes of zx and zy are the hyperbolas
| z2 | − | x2 | = 1, | z2 | − | y2 | = 1, |
| c2 | a2 | c2 | b2 |
having a common transverse axis along z′Oz; the section by any plane z = ±γ parallel to that of xy is the ellipse
| x2 | + | y2 | = | γ2 | − 1, |
| a2 | b2 | c2 |
provided γ2 > c2, and the surface, consisting of two distinct portions or sheets, may be considered as generated by a variable ellipse moving parallel to itself along the hyperbolas as directrices.
38. Differential Geometry of Curves.—For convenience consider the coordinates (x, y, z) of a point on a curve in space to be given as functions of a variable parameter θ, which may in particular be one of themselves. Use the notation x′, x″ for dx/dθ, d2x/dθ2, and similarly as to y and z. Only a few formulae will be given. Call the current coordinates (ξ, η, ζ).
The tangent at (x, y, z) is the line tended to as a limit by the connector of (x, y, z) and a neighbouring point of the curve when the latter moves up to the former: its equations are
The osculating plane at (x, y, z) is the plane tended to as a limit by that through (x, y, z) and two neighbouring points of the curve as these, remaining distinct, both move up to (x, y, z): its one equation is
The normal plane is the plane through (x, y, z) at right angles to the tangent line, i.e. the plane
It cuts the osculating plane in a line called the principal normal. Every line through (x, y, z) in the normal plane is a normal. The normal perpendicular to the osculating plane is called the binormal. A tangent, principal normal, and binormal are a convenient set of rectangular axes to use as those of reference, when the nature of a curve near a point on it is to be discussed.
Through (x, y, z) and three neighbouring points, all on the curve, passes a single sphere; and as the three points all move up to (x, y, z) continuing distinct, the sphere tends to a limiting size and position. The limit tended to is the sphere of closest contact with the curve at (x, y, z); its centre and radius are called the centre and radius of spherical curvature. It cuts the osculating plane in a circle, called the circle of absolute curvature; and the centre and radius of this circle are the centre and radius of absolute curvature. The centre of absolute curvature is the limiting position of the point where the principal normal at (x, y, z) is cut by the normal plane at a neighbouring point, as that point moves up to (x, y, z).
39. Differential Geometry of Surfaces.—Let (x, y, z) be any chosen point on a surface ƒ(x, y, z) = 0. As a second point of the surface moves up to (x, y, z), its connector with (x, y, z) tends to a limiting position, a tangent line to the surface at (x, y, z). All these tangent lines at (x, y, z), obtained by approaching (x, y, z) from different directions on a surface, lie in one plane
| ∂ƒ | (ξ − x) + | ∂ƒ | (η − y) + | ∂ƒ | (ζ − z) = 0. |
| ∂x | ∂y | ∂z |
This plane is called the tangent plane at (x, y, z). One line through (x, y, z) is at right angles to the tangent plane. This is the normal
| (ξ − x) / | ∂ƒ | = (η − y) / | ∂ƒ | = (ζ − z) / | ∂ƒ | . |
| ∂x | ∂y | ∂z |
The tangent plane is cut by the surface in a curve, real or imaginary, with a node or double point at (x, y, z). Two of the tangent lines touch this curve at the node. They are called the “chief tangents” (Haupt-tangenten) at (x, y, z); they have closer contact with the surface than any other tangents.
In the case of a quadric surface the curve of intersection of a tangent and the surface is of the second order and has a node, it must therefore consist of two straight lines. Consequently a quadric surface is covered by two sets of straight lines, a pair through every point on it; these are imaginary for the ellipsoid, hyperboloid of two sheets, and elliptic paraboloid.
A surface of any order is covered by two singly infinite systems of curves, a pair through every point, the tangents to which are all chief tangents at their respective points of contact. These are called chief-tangent curves; on a quadric surface they are the above straight lines.
40. The tangents at a point of a surface which bisect the angles between the chief tangents are called the principal tangents at the point. They are at right angles, and together with the normal constitute a convenient set of rectangular axes to which to refer the surface when its properties near the point are under discussion. At a special point which is such that the chief tangents there run to the circular points at infinity in the tangent plane, the principal tangents are indeterminate; such a special point is called an umbilic of the surface.
There are two singly infinite systems of curves on a surface, a pair cutting one another at right angles through every point upon it, all tangents to which are principal tangents of the surface at their respective points of contact. These are called lines of curvature, because of a property next to be mentioned.
As a point Q moves in an arbitrary direction on a surface from coincidence with a chosen point P, the normal at it, as a rule, at once fails to meet the normal at P; but, if it takes the direction of a line of curvature through P, this is instantaneously not the case. We have thus on the normal two centres of curvature, and the distances of these from the point on the surface are the two principal radii of curvature of the surface at that point; these are also the radii of curvature of the sections of the surface by planes through the normal and the two principal tangents respectively; or say they are the radii of curvature of the normal sections through the two principal tangents respectively. Take at the point the axis of z in the direction of the normal, and those of x and y in the directions of the principal tangents respectively, then, if the radii of curvature be a, b (the signs being such that the coordinates of the two centres of curvature are z = a and z = b respectively), the surface has in the neighbourhood of the point the form of the paraboloid
| z = | x2 | + | y2 | , |
| 2a | 2b |
and the chief-tangents are determined by the equation 0 = x22a + y22b. The two centres of curvature may be on the same side of the point or on opposite sides; in the former case a and b have the same sign, the paraboloid is elliptic, and the chief-tangents are imaginary; in the latter case a and b have opposite signs, the paraboloid is hyperbolic, and the chief-tangents are real.
The normal sections of the surface and the paraboloid by the same plane have the same radius of curvature; and it thence readily follows that the radius of curvature of a normal section of the surface by a plane inclined at an angle θ to that of zx is given by the equation
| 1 | = | cos2θ | + | sin2θ | . |
| ρ | a | b |
The section in question is that by a plane through the normal and a line in the tangent plane inclined at an angle θ to the principal tangent along the axis of x. To complete the theory, consider the section by a plane having the same trace upon the tangent plane, but inclined to the normal at an angle φ; then it is shown without difficulty (Meunier’s theorem) that the radius of curvature of this inclined section of the surface is = ρ cos φ.
Authorities.—The above article is largely based on that by Arthur Cayley in the 9th edition of this work. Of early and important recent publications on analytical geometry, special mention