co-ordinates; i.e. the positive directions of the axes are assumed
to be so arranged that a positive rotation of 90° about Ox would
bring Oy into the position of Oz, and so on. The displacement
will consist of an infinitesimal rotation ε about some axis through
O, whose direction-cosines are, say, l, m, n. From the equivalence
of a small rotation to a localized vector it follows that the
rotation ε will be equivalent to rotations ξ, η, ζ about Ox, Oy, Oz,
respectively, provided
and we note that
Thus in the case of fig. 36 it may be required to connect the infinitesimal rotations ξ, η, ζ about OA, OB, OC with the variations of the angular co-ordinates θ, ψ, φ. The displacement of the point C of the body is made up of δθ tangential to the meridian ZC and sin θ δψ perpendicular to the plane of this meridian. Hence, resolving along the tangents to the arcs BC, CA, respectively, we have
 |
| Fig. 40. |
Again, consider the point of the solid which was initially at A′ in the figure. This is displaced relatively to A′ through a space δψ perpendicular to the plane of the meridian, whilst A′ itself is displaced through a space cos θ δψ in the same direction. Hence
To find the component displacements of a point P of the body, whose co-ordinates are x, y, z, we draw PL normal to the plane yOz, and LH, LK perpendicular to Oy, Oz, respectively. The displacement of P parallel to Ox is the same as that of L, which is made up of ηz and −ζy. In this way we obtain the formulae
The most general case is derived from this by adding the component displacements λ, μ, ν (say) of the point which was at O; thus
δx = λ + ηz − ζy, |
The displacement is thus expressed in terms of the six independent
quantities ξ, η, ζ, λ, μ, ν. The points whose displacements
are in the direction of the resultant axis of rotation are
determined by δx : δy : δz = ξ : η : ζ, or
These are the equations of a straight line, and the displacement is in fact equivalent to a twist about a screw having this line as axis. The translation parallel to this axis is
The linear magnitude which measures the ratio of translation to rotation in a screw is called the pitch. In the present case the pitch is
Since ξ2 + η2 + ζ2, or ε2, is necessarily an absolute invariant for all transformations of the (rectangular) co-ordinate axes, we infer that λξ + μη + νζ is also an absolute invariant. When the latter invariant, but not the former, vanishes, the displacement is equivalent to a pure rotation.
If the small displacements of a rigid body be subject to one constraint, e.g. if a point of the body be restricted to lie on a given surface, the mathematical expression of this fact leads to a homogeneous linear equation between the infinitesimals ξ, η, ζ, λ, μ, ν, say
The quantities ξ, η, ζ, λ, μ, ν are no longer independent, and the body has now only five degrees of freedom. Every additional constraint introduces an additional equation of the type (10) and reduces the number of degrees of freedom by one. In Sir R. S. Ball’s Theory of Screws an analysis is made of the possible displacements of a body which has respectively two, three, four, five degrees of freedom. We will briefly notice the case of two degrees, which involves an interesting generalization of the method (already explained) of compounding rotations about intersecting axes. We assume that the body receives arbitrary twists about two given screws, and it is required to determine the character of the resultant displacement. We examine first the case where the axes of the two screws are at right angles and intersect. We take these as axes of x and y; then if ξ, η be the component rotations about them, we have
where h, k, are the pitches of the two given screws. The equations (7) of the axis of the resultant screw then reduce to
Hence, whatever the ratio ξ : η, the axis of the resultant screw lies on the conoidal surface
where c = 12(k − h). The co-ordinates of any point on (13) may be written
hence if we imagine a curve of sines to be traced on a circular cylinder so that the circumference just includes two complete undulations, a straight line cutting the axis of the cylinder at right angles and meeting this curve will generate the surface. This is called a cylindroid. Again, the pitch of the resultant screw is
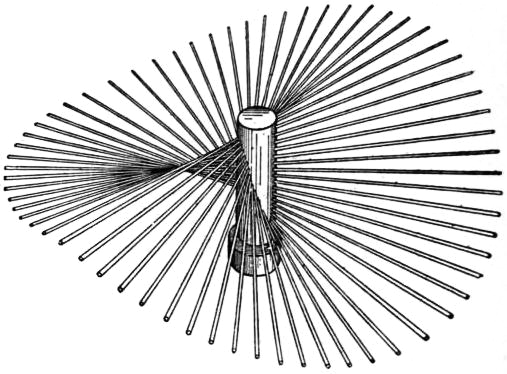 |
| From Sir Robert S. Ball’s Theory of Screws. |
| Fig. 41. |
The distribution of pitch among the various screws has therefore a simple relation to the pitch-conic
viz. the pitch of any screw varies inversely as the square of that diameter of the conic which is parallel to its axis. It is to be noticed that the parameter c of the cylindroid is unaltered if the two pitches h, k be increased by equal amounts; the only change is that all the pitches are increased by the same amount. It remains to show that a system of screws of the above type can be constructed so as to contain any two given screws whatever. In the first place, a cylindroid can be constructed so as to have its axis coincident with the common perpendicular to the axes of the two given screws and to satisfy three other conditions, for the position of the centre, the parameter, and the orientation about the axis are still at our disposal. Hence we can adjust these so that the surface shall contain the axes of the two given screws as generators, and that the difference of the corresponding pitches shall have the proper value. It follows that when a body has two degrees of freedom it can twist about any one of a singly infinite system of screws whose axes lie on a certain cylindroid. In particular cases the cylindroid may degenerate into a plane, the pitches being then all equal.
§ 8. Three-dimensional Statics.—A system of parallel forces can be combined two and two until they are replaced by a single resultant equal to their sum, acting in a certain line. As special cases, the system may reduce to a couple, or it may be in equilibrium.
In general, however, a three-dimensional system of forces cannot be replaced by a single resultant force. But it may be reduced to simpler elements in a variety of ways. For example, it may be reduced to two forces in perpendicular skew lines. For consider any plane, and let each force, at its intersection with the plane, be resolved into two components, one (P) normal to the plane, the other (Q) in the plane. The assemblage of parallel forces P can be replaced in general by a single force, and the coplanar system of forces Q by another single force.

