propagation of shad. The eggs are spawned in May and June, and are similar in the two species; they are heavier than the fresh water in which they develop, but unlike the herring's eggs they are not adhesive. They remain free and separate at the bottom of the river, carried down by the current or up by the tide. In the Elbe the twaite shad spawns below Hamburg, the allis shad above Dresden. In November the fry have reached 3 to 5 in. in length, but very few specimens in their second year have been found in rivers. The majority seem to descend to the sea before their first winter, to return when mature. On rivers in which these fishes make their periodical appearance they have become the object of a regular fishery. They are much esteemed on the middle Rhine, where they are generally known as “ Maifisch.” The allis shad is caught at a size from 15 to 24 in., and is better flavoured than the twaite shad, which is generally smaller.
Other, but closely allied species, occur on the Atlantic coasts of North America, all surpassing the European species in importance as food-fishes and economic value, viz., the American shad (Clupea sapidissima), the gaspereau or ale-wife (C. mattowocca or vernalis), and the menhaden (C. menhaden).
SHADDOCK (Citrus decumana), a tree allied to the orange
and the lemon, presumably native to the Malay and Polynesian
islands, but generally cultivated throughout the tropics. The
leaves are like those of the orange, but downy on the under
surface, as are also the young shoots. The flowers are large
and white, and are succeeded by very large globose fruits like
oranges, but paler in colour, and with a more pungent flavour.
The name Shaddock is asserted to be that of a captain who
introduced the tree to the West Indies. The fruit is also known
under the name of grape-fruit, pommeloes, and “forbidden fruit.”
Varieties occur with yellow and reddish pulp; and there are
also pear-shaped varieties.
SHADOOF (Arab. shdduf), an apparatus for drawing water,
used in the East generally, and particularly on the Nile for
the purpose of irrigation. It consists of an upright frame on
which is suspended a long pole at a distance of about one-fifth
of its length from one end; to the other end is attached a bucket
or skin bag, while at the short end a weight is suspended serving
as the counterpoise of a lever. The vessel containing the water
is then swung round and emptied into the runnel, which conveys
the water in the direction required.
SHADOW (O. Eng. Schadewe, sceadu; a form of “shade ”;
connected with Gr. axéfos, darkness). When an opaque body
is placed between a screen and a luminous source, it casts a
“ shadow ” on the screen. If the source be a point, such as the
image formed by a lens of small focus or by a fine hole in a plate
held close to a bright flame, the outline of the shadow is to be
found by drawing straight lines from the luminous point so as to
envelop the opaque body. These lines form a cone. The points
of contact form a line on the opaque body separating the
illuminated from the non-illuminated portion of its surface.
Similarly, when these lines are produced to meet the screen,
their points of intersection with it form a line which separates
the illuminated from the non-illuminated parts of the screen.
This line is called the boundary of the geometrical shadow, and
its construction is based on the assumption that light travels
in straight lines (in homogeneous media) and suffers no deviation
on meeting an obstacle. But a deviation, termed dijfraclion,
does occur, and consequently the complete theory of shadows
involves considerations based on the nature of the rays themselves;
this aspect is treated in DIFFRACTION or LIGHT. An
instance of the geometrical shadow is seen when a very small
gas-jet is burning in a ground-glass shade near a wall. In this
case the cone, above mentioned, is usually a right cone with its
axis vertical. Thus the boundary of the geometric shadow is a
portion of a circle on the roof, but a portion of an hyperbola
on the vertical wall. If the roof be not horizontal, we may obtain
in this way any form of conic section. Hints in projection may
be obtained by observing the shadows of bodies of various
forms cast in this way by rays which virtually diverge from one
point: e.g. how to place a plane quadrilateral of given form
so that its geometric shadow may be a square; how to place an
ellipticgdisk, with a small hole in it, so that the shadow may
be circular with a bright spot at its centre, &c.
When there are more luminous points than one, we have
only to draw separately the geometrical shadows due to each
of the sources, and then superpose them. A new consideration
now comes in. There will be, in general, portions of all the
separate geometrical shadows which overlap one another in some
particular regions of the screen. In such regions we still have
full shadow; but around them there will be other regions, some
illuminated by one of the sources alone, some by two, &c., until
finally we come to the parts of the screen which are illuminated
directly by all the sources. There will evidently be still a definite
boundary of the parts wholly unilluminated, 'i.e. the true shadow
or umbra, and also a dehnite boundary of the parts wholly
illuminated. The region between these boundaries-i.e. the
partially illumined portion-is called the penumbra.
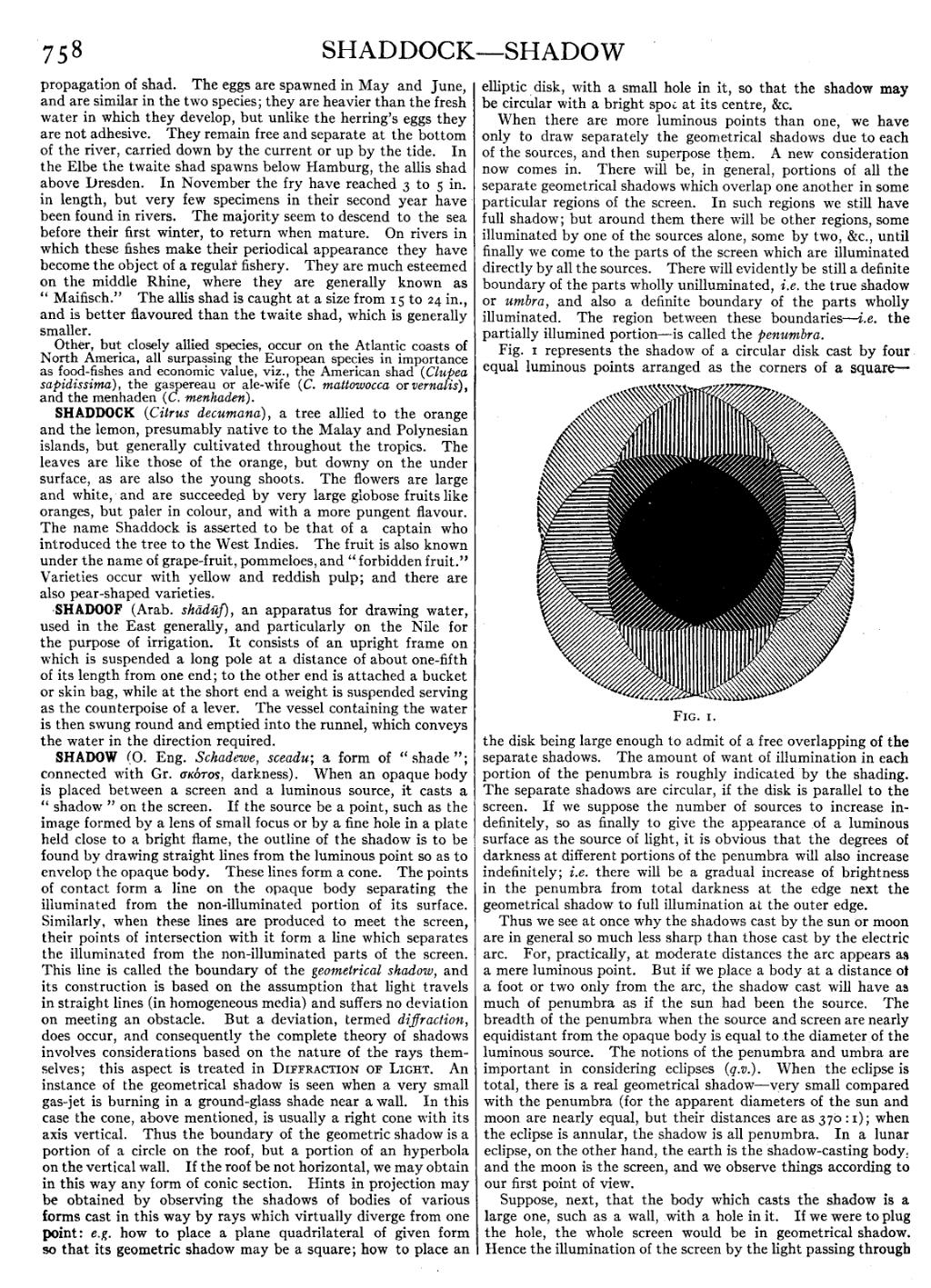
Fig. I represents the shadow of a circular disk cast by four
equal luminous points arranged as the corners of a square-FIG.
1.
the disk being large enough to admit of a free overlapping of the separate shadows. The amount of want of illumination in each portion of the penumbra is roughly indicated by the shading. The separate shadows are circular, if the disk is parallel to the screen. If we suppose the number of sources to increase indefinitely, so as finally to give the appearance, of a luminous surface as the source of light, it is obvious that the degrees of darkness at different portions of the penumbra will also increase indefinitely; i.e. there will be a gradual increase of brightness in the penumbra from total darkness at the edge next the geometrical shadow to full illumination at the outer edge. Thus we see at once why the shadows cast by the sun or moon are in general so much less sharp than those cast by the electric arc. For, practically, at moderate distances the arc appears as a mere luminous point. But if we place a body at a distance of a foot or two only from the arc, the shadow cast will have as much of penumbra as if the sun had been the source. The breadth of the penumbra when the source and screen are nearly equidistant from the opaque body is equal to the diameter of the luminous source. The notions of the penumbra and umbra are important in considering eclipses (q.11.). When the eclipse is total, there is a real geometrical shadow-very small compared with the penumbra (for the apparent diameters of the sun and moon are nearly equal, but their distances are as 370: 1); when the eclipse is annular, the shadow is all penumbra. In a lunar eclipse, on the other hand, the earth is the shadow-casting body, and the moon is the screen, and We observe things according to our first point of View.
Suppose, next, that the body which casts the shadow is a large one, such as a wall, with a hole init. If we were to plug the hole, the whole screen would be in geometrical shadow. Hence the illumination of the screen by the light passing through