On Physical Lines of Force
Part I: The Theory of Molecular Vortices applied to Magnetic Phenomena
[edit]
IN all phenomena involving attractions or repulsions, or any forces depending on the relative position of bodies, we have to determine the magnitude and direction of the force which would act on a given body, if placed in a given position.
In the case of a body acted on by the gravitation of a sphere, this force is inversely as the square of the distance, and in a straight line to the centre of the sphere. In the case of two attracting spheres, or of a body not spherical, the magnitude and direction of the force vary according to more complicated laws. In electric and magnetic phenomena, the magnitude and direction of the resultant force at any point is the main subject of investigation. Suppose that the direction of the force at any point is known, then, if we draw a line so that in every part of its course it coincides in direction with the force at that point, this line may be called a line of force, since it indicates the direction of the force in every part of its course.
By drawing a sufficient number of lines of force, we may indicate the direction of the force in every part of the space in which it acts.
Thus if we strew iron filings on paper near a magnet, each filing will be magnetized by induction, and the consecutive filings will unite by their opposite poles, so as to form fibres, and these fibres will indicate the direction of the lines of force. The beautiful illustration of the presence of magnetic force afforded by this experiment, naturally tends to make us think of the lines of force as something real, and as indicating something more than the mere resultant of two forces, whose seat of action is at a distance, and which do not exist there at all until a magnet is placed in that part of the field. We are dissatisfied with the explanation founded on the hypothesis of attractive and repellent forces directed towards the magnetic poles, even though we may have satisfied ourselves that the phenomenon is in strict accordance with that hypothesis, and we cannot help thinking that in every place where we find these lines of force, some physical state or action must exist in sufficient energy to produce the actual phenomena.
My object in this paper is to clear the way for speculation in this direction, by investigating the mechanical results of certain states of tension and motion in a medium, and comparing these with the observed phenomena of magnetism and electricity. By pointing out the mechanical consequences of such hypotheses, I hope to be of some use to those who consider the phenomena as due to the action of a medium, but are in doubt as to the relation of this hypothesis to the experimental laws already established, which have generally been expressed in the language of other hypotheses.
I have in a former paper[1] endeavoured to lay before the mind of the geometer a clear conception of the relation of the lines of force to the space in which they are traced. By making use of the conception of currents in a fluid, I showed how to draw lines of force, which should indicate by their number the amount of force, so that each line may be called a unit-line of force (see Faraday's `Researches,' 3122); and I have investigated the path of the lines where they pass from one medium to another.
In the same paper I have found the geometrical significance of the "Electrotonic State," and have shown how to deduce the mathematical relations between the electrotonic state, magnetism, electric currents, and the electromotive force, using mechanical illustrations to assist the imagination, but not to account for the phenomena.
I propose now to examine magnetic phenomena from a mechanical point of view, and to determine what tensions in, or motions of, a medium are capable of producing the mechanical phenomena observed. If, by the same hypothesis, we can connect the phenomena of magnetic attraction with electromagnetic phenomena and with those of induced currents, we shall have found a theory which, if not true, can only be proved to be erroneous by experiments which will greatly enlarge our knowledge of this part of physics.
The mechanical conditions of a medium under magnetic influence have been variously conceived of, as currents, undulations, or states of displacement or strain, or of pressure or stress.
Currents, issuing from the north pole and entering the south pole of a magnet, or circulating round an electric current, have the advantage of representing correctly the geometrical arrangement of the lines of force, if we could account on mechanical principles for the phenomena of attraction, or for the currents themselves, or explain their continued existence.
Undulations issuing from a centre would, according to the calculations of Professor Challis, produce an effect similar to attraction in the direction of the centre; but admitting this to be true, we know that two series of undulations traversing the same space do not combine into one resultant as two attractions do, but produce an effect depending on relations of phase as well as intensity, and if allowed to proceed, they diverge from each other without any mutual action. In fact the mathematical laws of attractions are not analogous in any respect to those of undulations, while they have remarkable analogies with those of currents, of the conduction of heat and electricity, and of elastic bodies.
In the Cambridge and Dublin Mathematical Journal for January 1847, Professor William Thomson has given a "Mechanical Representation of Electric, Magnetic, and Galvanic Forces," by means of the displacements of the particles of an elastic solid in a state of strain. In this representation we must make the angular displacement at every point of the solid proportional to the magnetic force at the corresponding point of the magnetic field, the direction of the axis of rotation of the displacement corresponding to the direction of the magnetic force. The absolute displacement of any particle will then correspond in magnitude and direction to that which I have identified with the electrotonic state; and the relative displacement of any particle, considered with reference to the particle in its immediate neighbourhood, will correspond in magnitude and direction to the quantity of electric current passing through the corresponding point of the magneto-electric field. The author of this method of representation does not attempt to explain the origin of the observed forces by the effects due to these strains in the elastic solid, but makes use of the mathematical analogies of the two problems to assist the imagination in the study of both.
We come now to consider the magnetic influence as existing in the form of some kind of pressure or tension, or, more generally, of stress in the medium.
Stress is action and reaction between the consecutive parts of a body, and consists in general of pressures or tensions different in different directions at the same point of the medium.
The necessary relations among these forces have been investigated by mathematicians; and it has been shown that the most general type of a stress consists of a combination of three principal pressures or tensions, in directions at right angles to each other.
When two of the principal pressures are equal, the third becomes an axis of symmetry, either of greatest or least pressure, the pressures at right angles to this axis being all equal.
When the three principal pressures are equal, the pressure is equal in every direction, and there results a stress having no determinate axis of direction, of which we have an example in simple hydrostatic pressure.
The general type of a stress is not suitable as a representation of a magnetic force, because a line of magnetic force has direction and intensity, but has no third quality indicating any difference between the sides of the line, which would be analogous to that observed in the case of polarized light[2].
We must therefore represent the magnetic force at a point by a stress having a single axis of greatest or least pressure, and all the pressures at right angles to this axis equal. It may be objected that it is inconsistent to represent a line of force, which is essentially dipolar, by an axis of stress, which is necessarily isotropic; but we know that every phenomenon of action and reaction is isotropic in its results, because the effects of the force on the bodies between which it acts are equal and opposite, while the nature and origin of the force may be dipolar, as in the attraction between a north and a south pole.
Let us next consider the mechanical effect of a state of stress symmetrical about an axis. We may resolve it, in all eases, into a simple hydrostatic pressure, combined with a simple pressure or tension along the axis. When the axis is that of greatest pressure, the force along the axis will be a pressure. When the axis is that of least pressure, the force along the axis will be a tension.
If we observe the lines of force between two magnets, as indicated by iron filings, we shall see that whenever the lines of force pass from one pole to another, there is attraction between those poles; and where the lines of force from the poles avoid each other and are dispersed into space, the poles repel each other, so that in both cases they are drawn in the direction of the resultant of the lines of force.
It appears therefore that the stress in the axis of a line of magnetic force is a tension, like that of a rope.
If we calculate the lines of force in the neighbourhood of two gravitating bodies, we shall find them the same in direction as those near two magnetic poles of the same name; but we know that the mechanical effect is that of attraction instead of repulsion. The lines of force in this case do not run between the bodies, but avoid each other, and are dispersed over space. In order to produce the effect of attraction, the stress along the lines of gravitating force must be a pressure.
Let us now suppose that the phenomena of magnetism depend on the existence of a tension in the direction of the lines of force, combined with a hydrostatic pressure; or in other words, a pressure greater in the equatorial than in the axial direction: the next question is, what mechanical explanation can we give of this inequality of pressures in a fluid or mobile medium? The explanation which most readily occurs to the mind is that the excess of pressure in the equatorial direction arises from the centrifugal force of vortices or eddies in the medium having their axes in directions parallel to the lines of force.
This explanation of the cause of the inequality of pressures at once suggests the means of representing the dipolar character of the line of force. Every vortex is essentially dipolar, the two extremities of its axis being distinguished by the direction of its revolution as observed from those points.
We also know that when electricity circulates in a conductor, it produces lines of magnetic force passing through the circuit, the direction of the lines depending on the direction of the circulation. Let us suppose that the direction of revolution of our vortices is that in which vitreous electricity must revolve in order to produce lines of force whose direction within the circuit is the same as that of the given lines of force.
We shall suppose at present that all the vortices in any one part of the field are revolving in the same direction about axes nearly parallel, but that in passing from one part of the field to another, the direction of the axes, the velocity of rotation, and the density of the substance of the vortices are subject to change. We shall investigate the resultant mechanical effect upon an element of the medium, and from the mathematical expression of this resultant we shall deduce the physical character of its different component parts.
Prop. I.—If in two fluid systems geometrically similar the velocities and densities at corresponding points are proportional, then the differences of pressure at corresponding points due to the motion will vary in the duplicate ratio of the velocities and the simple ratio of the densities.
Let l be the ratio of the linear dimensions, m that of the velocities, n that of the densities, and p that of the pressures due to the motion. Then the ratio of the masses of corresponding portions will be l3n, and the ratio of the velocities acquired in traversing similar parts of the systems will be ; so that is the ratio of the momenta acquired by similar portions in traversing similar parts of their paths.
The ratio of the surfaces is , that of the forces acting on them is , and that of the times during which they act is ; so that the ratio of the impulse of the forces is , and we have now
,
or
;
that is, the ratio of the pressures due to the motion is compounded of the ratio of the densities and the duplicate ratio of the velocities , and does not depend on the linear dimensions of the moving systems.
In a circular vortex, revolving with uniform angular velocity, if the pressure at the axis is , that at the circumference will be , where is the density and the velocity at the circumference. The mean pressure parallel to the axis will be
.
If a number of such vortices were placed together side by side with their axes parallel, they would form a medium in which there would be a pressure parallel to the axes, and a pressure in any perpendicular direction. If the vortices are circular, and have uniform angular velocity and density throughout, then
.
If the vortices are not circular, and if the angular velocity and the density are not uniform, but vary according to the same law for all the vortices,
,
where is the mean density, and is a numerical quantity depending on the distribution of angular velocity and density in the vortex. In future we shall write instead of , so that
where is a quantity bearing a constant ratio to the density, and is the linear velocity at the circumference of each vortex.
A medium of this kind, filled with molecular vortices having their axes parallel, differs from an ordinary fluid in having different pressures in different directions. If not prevented by properly arranged pressures, it would tend to expand laterally. In so doing, it would allow the diameter of each vortex to expand and its velocity to diminish in the same proportion. In order that a medium having these inequalities of pressure in different directions should be in equilibrium, certain conditions must be fulfilled, which we must investigate.
Prop. II. — If the direction-cosines of the axes of the vortices with respect to the axes of x, y, and z be l, m, and n, to find the normal and tangential stresses on the coordinate planes.
The actual stress may be resolved into a simple hydrostatic pressure acting in all directions, and a simple tension , or ,acting along the axis of stress.
Hence if , , and be the normal stresses parallel to the three axes, considered positive when they tend to increase those axes; and if , , and be the tangential stresses in the three coordinate planes, considered positive when they tend to increase simultaneously the symbols subscribed, then by the resolution of stresses[3],
If we write
then
| (2) |
Prop. III.—To find the resultant force on an element of the medium, arising from the variation of internal stress.
We have in general, for the force in the direction of per unit of volume by the law of equilibrium of stresses[4],
| (3) |
In this case the expression may be written
| (4) |
Remembering that , this becomes
| (5) |
The expressions for the forces parallel to the axes of and may be written down from analogy.
We have now to interpret the meaning of each term of this expression.
We suppose to be the components of the force which would act upon that end of a unit magnetic bar which points to the north.
represents the magnetic inductive capacity of the medium at any point referred to air as a standard. represent the quantity of magnetic induction through unit of area perpendicular to the three axes of respectively.
The total amount of magnetic induction through a closed surface surrounding the pole of a magnet, depends entirely on the strength of that pole; so that if dx dy dz be an element, then
| (6) |
which represents the total amount of magnetic induction outwards through the surface of the element dx dy dz, represents the amount of "imaginary magnetic matter" within the element, of the kind which points north.
The first term of the value of , therefore,
| (7) |
may be written
| (8) |
The physical interpretation of this term is, that the force urging a north pole in the positive direction of is the product of the intensity of the magnetic force resolved in that direction, and the strength of the north pole of the magnet.
Let the parallel lines from left to right in fig. 1 represent a field of magnetic force such as that of the earth, being the direction from south to north. The vortices, according to our hypothesis, will be in the direction shown by the arrows in fig. 3, that is, in a plane perpendicular to the lines of force, and revolving in the direction of the hands of a watch when observed from looking towards . The parts of the vortices above the plane of the paper will be moving towards , and the parts below that plane towards .
We shall always mark by an arrow-head the direction in which we must look in order to see the vortices rotating in the direction of the hands of a watch. The arrow-head will then indicate the northward direction in the magnetic field, that is, the direction in which that end of a magnet which points to the north would set itself in the field.
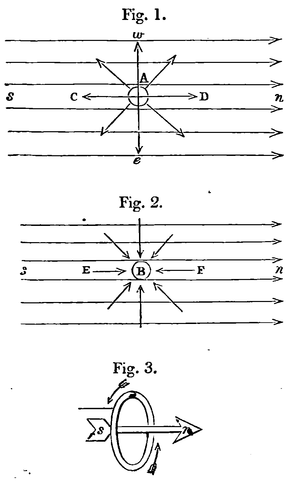
Now let A be the end of a magnet which points north. Since it repels the north ends of other magnets, the lines of force will be directed from A outwards in all directions. On the north side the line AD will be in the same direction with the lines of the magnetic field, and the velocity of the vortices will he increased. On the south side the line AC will be in the opposite direction, and the velocity of the vortices will be diminished, so that the lines of force are more powerful on the north side of A than on the south side.
We have seen that the mechanical effect of the vortices is to produce a tension along their axes, so that the resultant effect on A will be to pull it more powerfully towards D than towards C; that is, A will tend to move to the north.
Let B in fig. 2 represent a south pole. The lines of force belonging to B will tend towards B, and we shall find that the lines of force are rendered stronger towards E than towards F, so that the effect in this case is to urge B towards the south.
It appears therefore that, on the hypothesis of molecular vortices, our first term gives a mechanical explanation of the force acting on a north or south pole in the magnetic field.
We now proceed to examine the second term,
Here is the square of the intensity at any part of the field, and is the magnetic inductive capacity at the same place. Any body therefore placed in the field will be urged towards places of stronger magnetic intensity with, a force depending partly on its own capacity for magnetic induction, and partly on the rate at which the square of the intensity increases.
If the body be placed in a fluid medium, then the medium, as well as the body, will be urged towards places of greater intensity, so that its hydrostatic pressure will be increased in that direction. The resultant effect on a body placed in the medium will be the difference of the actions on the body and on the portion of the medium which it displaces, so that the body will tend to or from places of greatest magnetic intensity, according as it has a greater or less capacity for magnetic induction than the surrounding medium.
In fig. 4 the lines of force are represented as converging and becoming more powerful towards the right, so that the magnetic tension at B is stronger than at A, and the body AB will be urged to the right. If the capacity for magnetic induction is greater in the body than in the surrounding medium, it will move to the right, but if less it will move to the left.

We may suppose in this case that the lines of force are converging to a magnetic pole, either north or south, on the right hand.
In fig. 5 the lines of force are represented as vertical, and becoming coming more numerous towards the right. It may be shown that if the force increases towards the right, the lines of force will be curved towards the right. The effect of the magnetic tensions will then be to draw any body towards the right with a force depending on the excess of its inductive capacity over that of the surrounding medium.
We may suppose that in this figure the lines of force are those surrounding an electric current perpendicular to the plane of the paper and on the right hand of the figure.
These two illustrations will show the mechanical effect on a paramagnetic or diamagnetic body placed in a field of varying magnetic force, whether the increase of force takes place along the lines or transverse to them. The form of the second term of our equation indicates the general law, which is quite independent of the direction of the lines of force, and depends solely on the manner in which the force varies from one part of the field to another.
We come now to the third term of the value of X,
Here is, as before, the quantity of magnetic induction through unit of area perpendicular to the axis of , and is a quantity which would disappear if were a complete differential, that is, if the force acting on a unit north pole were subject to the condition that no work can be done upon the pole in passing round any closed curve. The quantity represents the work done on a north pole in travelling round unit of area in the direction from + to + parallel to the plane of . Now if an electric current whose strength is is traversing the axis of , which, we may suppose, points vertically upwards, then, if the axis of is east and that of north, a unit north pole will be urged round the axis of in the direction from to , so that in one revolution the work done will be . Hence represents the strength of an electric current parallel to z through unit of area; and if we write
| (9) |
then p, q, r will be the quantity of electric current per unit of area perpendicular to the axes of x, y, and respectively.
The physical interpretation of the third term of X, , is that if is the quantity of magnetic induction parallel to , and the quantity of electricity flowing in the direction of , the element will be urged in the direction of -, transversely to the direction of the current and of the lines of force; that is, an ascending current in a field of force magnetized towards the north would tend to move west.

To illustrate the action of the molecular vortices, let be the direction of magnetic force in the field, and let C be the section of an ascending magnetic current perpendicular to the paper. The lines of force due to this current will be circles drawn in the opposite direction from that of the hands of a watch; that is, in the direction . At the lines of force will be the sum of those of the field and of the current, and at they will be the difference of the two sets of lines; so that the vortices on the east side of the current will be more powerful than those on the west side. Both sets of vortices have their equatorial parts turned towards C, so that they tend to expand towards C, but those on the east side have the greatest effect, so that the resultant effect on the current is to urge it towards the west.
The fourth term,
| (10) |
may be interpreted in the same way, and indicates that a current in the direction of , that is, to the north, placed in a magnetic field in which the lines are vertically upwards in the direction of , will be urged towards the east.
The fifth term,
| (11) |
merely implies that the element will be urged in the direction in which the hydrostatic pressure diminishes.
We may now write down the expressions for the components of the resultant force on an element of the medium per unit of volume, thus:
| (12) |
| (13) |
| (14) |
The first term of each expression refers to the force acting on magnetic poles.
The second term to the action on bodies capable of magnetism by induction.
The third and fourth terms to the force acting on electric currents.
And the fifth to the effect of simple pressure.
Before going further in the general investigation, we shall consider equations (12, 13, 14,) in particular cases, corresponding to those simplified cases of the actual phenomena which we seek to obtain in order to determine their laws by experiment.
We have found that the quantities p, q, and represent the resolved parts of an electric current in the three coordinate directions. Let us suppose in the first instance that there is no electric current, or that p, q, and vanish. We have then by (9),
| (15) |
whence we learn that
| (16) |
is an exact differential of , so that
| (17) |
is proportional to the density of the vortices, and represents the "capacity for magnetic induction" in the medium. It is equal to 1 in air, or in whatever medium the experiments were made which determined the powers of the magnets, the strengths of the electric currents, &c.
Let us suppose constant, then
| (18) |
represents the amount of imaginary magnetic matter in unit of volume. That there may be no resultant force on that unit of volume arising from the action represented by the first term of equations (12, 13, 14), we must have , or
| (19) |
Now it may be shown that equation (19), if true within a given space, implies that the forces acting within that space are such as would result from a distribution of centres of force beyond that space, attracting or repelling inversely as the square of the distance.
Hence the lines of force in a part of space where is uniform, and where there are no electric currents, must be such as would result from the theory of "imaginary matter" acting at a distance. The assumptions of that theory are unlike those of ours, but the results are identical.
Let us first take the case of a single magnetic pole, that is, one end of a long magnet, so long that its other end is too far off to have a perceptible influence on the part of the field we are considering. The conditions then are, that equation (18) must be fulfilled at the magnetic pole, and (19) everywhere else. The only solution under these conditions is
| (20) |
where is the distance from the pole, and the strength of the pole.
The repulsion at any point on a unit pole of the same kind is
| (21) |
In the standard medium ; so that the repulsion is simply in that medium, as has been shown by Coulomb.
In a medium having a greater value of (such as oxygen, solutions of salts of iron, &c.) the attraction, on our theory, ought to be less than in air, and in diamagnetic media (such as water, melted bismuth, &c.) the attraction between the same magnetic poles ought to be greater than in air.
The experiments necessary to demonstrate the difference of attraction of two magnets according to the magnetic or diamagnetic character of the medium in which they are placed, would require great precision, on account of the limited range of magnetic capacity in the fluid media known to us, and the small amount of the difference sought for as compared with the whole attraction.
Let us next take the case of an electric current whose quantity is C, flowing through a cylindrical conductor whose radius is R, and whose length is infinite as compared with the size of the field of force considered.
Let the axis of the cylinder be that of , and the direction of the current positive, then within the conductor the quantity of current per unit of area is
| (22) |
| (23) |
Beyond the conductor, in the space round it,
| (24) |
| (25) |
If is the perpendicular distance of any point from the axis of the conductor, a unit north pole will experience a force , tending to move it round the conductor in the direction of the hands of a watch, if the observer view it in the direction of the current.
Let us now consider a current running parallel to the axis of in the plane of at a distance . Let the quantity of the current be , and let the length of the part considered be , and its section , so that is its strength per unit of section. Putting this quantity for in equations (12, 13, 14), we find
per unit of volume; and multiplying by , the volume of the conductor considered, we find
| (26) |
showing that the second conductor will be attracted towards the first with a force inversely as the distance.
We find in this case also that the amount of attraction depends on the value of , but that it varies directly instead of inversely as ; so that the attraction between two conducting wires will be greater in oxygen than in air, and greater in air than in water.
We shall next consider the nature of electric currents and electromotive forces in connexion with the theory of molecular vortices.
Part II: The Theory of Molecular Vortices applied to Electric Currents
[edit]
WE have already shown that all the forces acting between magnets, substances capable of magnetic induction, and electric currents, may be mechanically accounted for on the supposition that the surrounding medium is put into such a state that at every point the pressures are different in different directions, the direction of least pressure being that of the observed lines of force, and the difference of greatest and least pressures being proportional to the square of the intensity of the force at that point.
Such a state of stress, if assumed to exist in the medium, and to be arranged according to the known laws regulating lines of force, will act upon the magnets, currents, &c. in the field with precisely the same resultant forces as those calculated on the ordinary hypothesis of direct action at a distance. This is true independently of any particular theory as to the cause of this state of stress, or the mode in which it can be sustained in the medium. We have therefore a satisfactory answer to the question, "Is there any mechanical hypothesis as to the condition of the medium indicated by lines of force, by which the observed resultant forces may be accounted for?" The answer is, the lines of force indicate the direction of minimum pressure at every point of the medium.
The second question must be, "What is the mechanical cause of this difference of pressure in different directions?" We have supposed, in the first part of this paper, that this difference of pressures is caused by molecular vortices, having their axes parallel to the lines of force.
We also assumed, perfectly arbitrarily, that the direction of these vortices is such that, on looking along a line of force from south to north, we should see the vortices revolving in the direction of the hands of a watch.
We found that the velocity of the circumference of each vortex must be proportional to the intensity of the magnetic force, and that the density of the substance of the vortex must be proportional to the capacity of the medium for magnetic induction.
We have as yet given no answers to the questions, "How are these vortices set in rotation?" and "Why are they arranged according to the known laws of lines of force about magnets and currents?" These questions are certainly of a higher order of difficulty than either of the former; and I wish to separate the suggestions I may offer by way of provisional answer to them, from the mechanical deductions which resolved the first question, and the hypothesis of vortices which gave a probable answer to the second.
We have, in fact, now come to inquire into the physical connexion of these vortices with electric currents, while we are still in doubt as to the nature of electricity, whether it is one substance, two substances, or not a substance at all, or in what way it differs from matter, and how it is connected with it.

I have found great difficulty in conceiving of the existence of vortices in a medium, side by side, revolving in the same direction about parallel axes. The contiguous portions of consecutive vortices must be moving in opposite directions; and it is difficult to understand how the motion of one part of the medium can coexist with, and even produce, an opposite motion of a part in contact with it.
The only conception which has at all aided me in conceiving of this kind of motion is that of the vortices being separated by a layer of particles, revolving each on its own axis in the opposite direction to that of the vortices, so that the contiguous surfaces of the particles and of the vortices have the same motion.
In mechanism, when two wheels are intended to revolve in the same direction, a wheel is placed between them so as to be in gear with both, and this wheel is called an "idle wheel." The hypothesis about the vortices which I have to suggest is that a layer of particles, acting as idle wheels, is interposed between each vortex and the next, so that each vortex has a tendency to make the neighbouring vortices revolve in the same direction with itself.
In mechanism, the idle wheel is generally made to rotate about a fixed axle; but in epicyclic trains and other contrivances, as, for instance, in Siemens's governor for steam-engines[5], we find idle wheels whose centres are capable of motion. In all these cases the motion of the centre is the half sum of the motions of the circumferences of the wheels between which it is placed. Let us examine the relations which must subsist between the motions of our vortices and those of the layer of particles interposed as idle wheels between them.
Prop. IV. — To determine the motion of a layer of particles separating two vortices.
Let the circumferential velocity of a vortex, multiplied by the three direction-cosines of its axis respectively, be α, β, γ, as in Prop. II. Let l, m, n be the direction-cosines of the normal to any part of the surface of this vortex, the outside of the surface being regarded positive. Then the components of the velocity of the particles of the vortex at this part of its surface will be
If this portion of the surface be in contact with another vortex whose velocities are , then a layer of very small particles placed between them will have a velocity which will be the mean of the superficial velocities of the vortices which they separate, so that if is the velocity of the particles in the direction of ,
| (27) |
since the normal to the second vortex is in the opposite direction to that of the first.
Prop. V.— To determine the whole amount of particles transferred across unit of area in the direction of in unit of time.
Let be the coordinates of the centre of the first vortex, those of the second, and so on. Let , &c. be the volumes of the first, second, &c. vortices, and the sum of their volumes. Let be an element of the surface separating the first and second vortices, and x, y, z its coordinates. Let be the quantity of particles on every unit of surface. Then if be the whole quantity of particles transferred across unit of area in unit of time in the direction of , the whole momentum parallel to of the particles within the space whose volume is will be , and we shall have
| (28) |
the summation being extended to every surface separating any two vortices within the volume .
Let us consider the surface separating the first and second vortices. Let an element of this surface be , and let its direction-cosines be with respect to the first vortex, and with respect to the second; then we know that
| (29) |
The values of vary with the position of the centre of the vortex; so that we may write
| (30) |
with similar equations for and .
The value of may be written:—
| (31) |
In effecting the summation of , we must remember that round any closed surface and all similar terms vanish; also that terms of the form , where and are measured in different directions, also vanish; but that terms of the form , where and refer to the same axis of coordinates, do not vanish, but are equal to the volume enclosed by the surface. The result is
| (32) |
or dividing by ,
| (33) |
If we make
| (34) |
then equation (33) will be identical with the first of equations (9), which give the relation between the quantity of an electric current and the intensity of the lines of force surrounding it.
It appears therefore that, according to our hypothesis, an electric current is represented by the transference of the moveable particles interposed between the neighbouring vortices. We may conceive that these particles are very small compared with the size of a vortex, and that the mass of all the particles together is inappreciable compared with that of the vortices, and that a great many vortices, with their surrounding particles, are contained in a single complete molecule of the medium. The particles must be conceived to roll without sliding between the vortices which they separate, and not to touch each other, so that, as long as they remain within the same complete molecule, there is no loss of energy by resistance. When, however, there is a general transference of particles in one direction, they must pass from one molecule to another, and in doing so, may experience resistance, so as to waste electrical energy and generate heat.
Now let us suppose the vortices arranged in a medium in any arbitrary manner. The quantities , &c. will then in general have values, so that there will at first be electrical currents in the medium. These will be opposed by the electrical resistance of the medium; so that, unless they are kept up by a continuous supply of force, they will quickly disappear, and we shall then have , &c.; that is, will be a complete differential (see equations (15) and (16)); so that our hypothesis accounts for the distribution of the lines of force.
In Plate V. fig. 1, let the vertical circle EE represent an electric current flowing from copper C to zinc Z through the conductor EE', as shown by the arrows.
Let the horizontal circle MM' represent a line of magnetic force embracing the electric circuit, the north and south directions being indicated by the lines SN and NS.
Let the vertical circles V and V' represent the molecular vortices of which the line of magnetic force is the axis. V revolves as the hands of a watch, and V the opposite way.
It will appear from this diagram, that if V and V' were contiguous vortices, particles placed between them would move downwards; and that if the particles were forced downwards by any cause, they would make the vortices revolve as in the figure. We have thus obtained a point of view from which we may regard the relation of an electric current to its lines of force as analogous to the relation of a toothed wheel or rack to wheels which it drives.
In the first part of the paper we investigated the relations of the statical forces of the system. We have now considered the connexion of the motions of the parts considered as a system of mechanism. It remains that we should investigate the dynamics of the system, and determine the forces necessary to produce given changes in the motions of the different parts.
Prop. VI.— To determine the actual energy of a portion of a medium due to the motion of the vortices within it.
Let be the components of the circumferential velocity, as in Prop. II., then the actual energy of the vortices in unit of volume will be proportional to the density and to the square of the velocity. As we do not know the distribution of density and velocity in each vortex, we cannot determine the numerical value of the energy directly; but since also bears a constant though unknown ratio to the mean density, let us assume that the energy in unit of volume is
where C is a constant to be determined.
Let us take the case in which
| (35) |
Let
| (36) |
and let
| (37) |
then is the potential at any point due to the magnetic system , and that due to the distribution of magnetism represented by . The actual energy of all the vortices is
| (38) |
the integration being performed over all space.
This may be shown by integration by parts (see Green's 'Essay on Electricity,' p. 10) to be equal to
| (39) |
Or since it has been proved (Green's 'Essay,' p. 10) that
| (40) |
Now let the magnetic system remain at rest, and let be moved parallel to itself in the direction of through a space ; then, since depends on only, it will remain as before, so that will be constant; and since depends on only, the distribution of about will remain the same, so that will be the same as before the change. The only part of E that will be altered is that depending on , because becomes on account of the displacement. The variation of actual energy due to the displacement is therefore
| (41) |
But by equation (12), the work done by the mechanical forces on during the motion is
| (42) |
and since our hypothesis is a purely mechanical one, we must have by the conservation of force,
| (43) |
that is, the loss of energy of the vortices must be made up by work done in moving magnets, so that
or
| (44) |
so that the energy of the vortices in unit of volume is
| (45) |
and that of a vortex whose volume is V is
| (46) |
In order to produce or destroy this energy, work must be expended on, or received from, the vortex, either by the tangential action of the layer of particles in contact with it, or by change of form in the vortex. We shall first investigate the tangential action between the vortices and the layer of particles in contact with them.
Prop. VII. — To find the energy spent upon a vortex in unit of time by the layer of particles which surrounds it.
Let P, Q, R be the forces acting on unity of the particles in the three coordinate directions, these quantities being functions of x, y, and z. Since each particle touches two vortices at the extremities of a diameter, the reaction of the particle on the vortices will be equally divided, and will be
on each vortex for unity of the particles; but since the superficial density of the particles is (see equation (34)), the forces on unit of surface of a vortex will be
Now let be an element of the surface of a vortex. Let the direction-cosines of the normal be l, m, n. Let the coordinates of the element be x, y, z. Let the component velocities of the surface be u, v, w. Then the work expended on that clement of surface will be
| (47) |
Let us begin with the first term, . may be written
| (48) |
and
Remembering that the surface of the vortex is a closed one, so that
and
we find
| (49) |
and the whole work done on the vortex in unit of time will be
| (50) |
Prop. VIII.— To find the relations between the alterations of motion of the vortices, and the forces P, Q, R which they exert on the layer of particles between them.
Let V be the volume of a vortex, then by (46) its energy is
| (51) |
and
| (52) |
Comparing this value with that given in equation (50), we find
| (53) |
This equation being true for all values of , and , first let and vanish, and divide by . We find
| (54) |
From these equations we may determine the relation between the alterations of motion , &c. and the forces exerted on the layers of particles between the vortices, or, in the language of our hypothesis, the relation between changes in the state of the magnetic field and the electromotive forces thereby brought into play.
In a memoir "On the Dynamical Theory of Diffraction" (Cambridge Philosophical Transactions, vol. ix. part 1, section 6), Professor Stokes has given a method by which we may solve equations (54), and find P, Q, and R in terms of the quantities on the right-hand of those equations. I have pointed out[6] the application of this method to questions in electricity and magnetism.
Let us then find three quantities F, G, H from the equations
| (55) |
with the conditions
| (56) |
and
| (57) |
Differentiating (55) with respect to , and comparing with (54), we find
| (58) |
We shall in the first place examine the process by which the lines of force are produced by an electric current.
Let AB, Pl. V. fig. 2, represent a current of electricity in the direction from A to B. Let the large spaces above and below AB represent the vortices, and let the small circles separating the vortices represent the layers of particles placed between them, which in our hypothesis represent electricity.
Now let an electric current from left to right commence in AB. The row of vortices above AB will be set in motion in the opposite direction to that of a watch. (We shall call this direction +, and that of a watch -.) We shall suppose the row of vortices still at rest, then the layer of particles between these rows will be acted on by the row on their lower sides, and will be at rest above. If they are free to move, they will rotate in the negative direction, and will at the same time move from right to left, or in the opposite direction from the current, and so form an induced electric current.
If this current is checked by the electrical resistance of the medium, the rotating particles will act upon the row of vortices , and make them revolve in the positive direction till they arrive at such a velocity that the motion of the particles is reduced to that of rotation, and the induced current disappears. If, now, the primary current be stopped, the vortices in the row will be checked, while those of the row still continue in rapid motion. The momentum of the vortices beyond the layer of particles will tend to move them from left to right, that is, in the direction of the primary current; but if this motion is resisted by the medium, the motion of the vortices beyond will be gradually destroyed.
It appears therefore that the phenomena of induced currents are part of the process of communicating the rotatory velocity of the vortices from one part of the field to another.
As an example of the action of the vortices in producing induced currents, let us take the following case:—Let B, Pl. V. fig. 3, be a circular ring, of uniform section, lapped uniformly with covered wire. It may be shown that if an electric current is passed through this wire, a magnet placed within the coil of wire will be strongly affected, but no magnetic effect will be produced on any external point. The effect will be that of a magnet bent round till its two poles are in contact.
If the coil is properly made, no effect on a magnet placed outside it can be discovered, whether the current is kept constant or made to vary in strength; but if a conducting wire C be made to embrace the ring any number of times, an electromotive force will act on that wire whenever the current in the coil is made to vary; and if the circuit be closed, there will be an actual current in the wire C.
This experiment shows that, in order to produce the electromotive force, it is not necessary that the conducting wire should be placed in a field of magnetic force, or that lines of magnetic force should pass through the substance of the wire or near it. All that is required is that lines of force should pass through the circuit of the conductor, and that these lines of force should vary in quantity during the experiment.
In this case the vortices, of which we suppose the lines of magnetic force to consist, are all within the hollow of the ring, and outside the ring all is at rest. If there is no conducting circuit embracing the ring, then, when the primary current is made or broken, there is no action outside the ring, except an instantaneous pressure between the particles and the vortices which they separate. If there is a continuous conducting circuit embracing the ring, then, when the primary current is made, there will be a current in the opposite direction through C; and when it is broken, there will be a current through C in the same direction as the primary current.
We may now perceive that induced currents are produced when the electricity yields to the electromotive force, — this force, however, still existing when the formation of a sensible current is prevented by the resistance of the circuit.
The electromotive force, of which the components are P, Q, R, arises from the action between the vortices and the interposed particles, when the velocity of rotation is altered in any part of the field. It corresponds to the pressure on the axle of a wheel in a machine when the velocity of the driving wheel is increased or diminished.
The electrotonic state, whose components are F, G, H, is what the electromotive force would be if the currents, &c. to which the lines of force are due, instead of arriving at their actual state by degrees, had started instantaneously from rest with their actual values. It corresponds to the impulse which would act on the axle of a wheel in a machine if the actual velocity were suddenly given to the driving wheel, the machine being previously at rest.
If the machine were suddenly stopped by stopping the driving wheel, each wheel would receive an impulse equal and opposite to that which it received when the machine was set in motion.
This impulse may be calculated for any part of a system of mechanism, and may be called the reduced momentum of the machine for that point. In the varied motion of the machine, the actual force on any part arising from the variation of motion may be found by differentiating the reduced momentum with respect to the time, just as we have found that the electromotive force may be deduced from the electrotonic state by the same process.
Having found the relation between the velocities of the vortices and the electromotive forces when the centres of the vortices are at rest, we must extend our theory to the case of a fluid medium containing vortices, and subject to all the varieties of fluid motion. If we fix our attention on any one elementary portion of a fluid, we shall find that it not only travels from one place to another, but also changes its form and position, so as to be elongated in certain directions and compressed in others, and at the same time (in the most general case) turned round by a displacement of rotation.
These changes of form and position produce changes in the velocity of the molecular vortices, which we must now examine.
The alteration of form and position may always be reduced to three simple extensions or compressions in the direction of three rectangular axes, together with three angular rotations about any set of three axes. We shall first consider the effect of three simple extensions or compressions.
Prop. IX. — To find the variations of in the parallelopiped x, y, z when becomes ; , ; and , , the volume of the figure remaining the same.
By Prop. II. we find for the work done by the vortices against pressure,
| (59) |
and by Prop. VI. we find for the variation of energy,
| (60) |
The sum must be zero by the conservation of energy, and , since is constant; so that
| (61) |
In order that this should be true independently of any relations between , , and , we must have
| (62) |
Prop. X. — To find the variations of due to a rotation about the axis of from to , a rotation about the axis of from to , and a rotation about the axis of from to .
The axis of will move away from the axis of by an angle , so that resolved in the direction of changes from 0 to .
The axis of approaches that of by an angle ; so that the resolved part of in direction changes from 0 to .
The resolved part of a. in the direction of changes by a quantity depending on the second power of the rotations, which may be neglected. The variations of from this cause are therefore
| (63) |
The most general expressions for the distortion of an element produced by the displacement of its different parts depend on the nine quantities
and these may always be expressed in terms of nine other quantities, namely, three simple extensions or compressions,
Let the direction-cosines of with respect to x, y, z be , those of and those of ; then we find
| (64) |
with similar equations for quantities involving and .
Let be the values of referred to the axes of ; then
| (65) |
We shall then have
| (66) |
| (67) |
By substituting the values of , and comparing with equations (64), we find
| (68) |
as the variation of due to the change of form and position of the element. The variations of and have similar expressions.
Prop. XI. — To find the electromotive forces in a moving body.
The variation of the velocity of the vortices in a moving element is due to two causes — the action of the electromotive forces, and the change of form and position of the element. The whole variation of is therefore
| (69) |
But since a is a function of x, y, z and t, the variation of may be also written
| (70) |
| (71) |
and that in the absence of free magnetism
| (72) |
we find
| (73) |
Putting
| (74) |
and
| (75) |
where and are the values of the electrotonic components for a fixed point of space, our equation becomes
| (76) |
The expressions for the variations of and give us two other equations which may be written down from symmetry. The complete solution of the three equations is
| (77) |
The first and second terms of each equation indicate the effect of the motion of any body in the magnetic field, the third term refers to changes in the electrotonic state produced by alterations of position or intensity of magnets or currents in the field, and is a function of and , which is indeterminate as far as regards the solution of the original equations, but which may always be determined in any given case from the circumstances of the problem. The physical interpretation of is, that it is the electric tension at each point of space.
The physical meaning of the terms in the expression for the electromotive force depending on the motion of the body, may be made simpler by supposing the field of magnetic force uniformly magnetized with intensity in the direction of the axis of . Then if l, m, n be the direction-cosines of any portion of a linear conductor, and S its length, the electromotive force resolved in the direction of the conductor will be
| (78) |
or
| (79) |
that is, the product of , the quantity of magnetic induction over unit of area multiplied by , the area swept out by the conductor in unit of time, resolved perpendicular to the direction of the magnetic force.
The electromotive force in any part of a conductor due to its motion is therefore measured by the number of lines of magnetic force which it crosses in unit of time; and the total electromotive force in a closed conductor is measured by the change of the number of lines of force which pass through it; and this is true whether the change be produced by the motion of the conductor or by any external cause.
In order to understand the mechanism by which the motion of a conductor across lines of magnetic force generates an electromotive force in that conductor, we must remember that in Prop. X. we have proved that the change of form of a portion of the medium containing vortices produces a change of the velocity of those vortices; and in particular that an extension of the medium in the direction of the axes of the vortices, combined with a contraction in all directions perpendicular to this, produces an increase of velocity of the vortices; while a shortening of the axis and bulging of the sides produces a diminution of the velocity of the vortices.
This change of the velocity of the vortices arises from the internal effects of change of form, and is independent of that produced by external electromotive forces. If, therefore, the change of velocity be prevented or checked, electromotive forces will arise, because each vortex will press on the surrounding particles in the direction in which it tends to alter its motion.
Let A, fig. 4, represent the section of a vertical wire moving in the direction of the arrow from west to east, across a system of lines of magnetic force running north and south. The curved lines in fig. 4 represent the lines of fluid motion about the wire, the wire being regarded as stationary, and the fluid as having a motion relative to it. It is evident that, from this figure, we can trace the variations of form of an element of the fluid, as the form of the element depends, not on the absolute motion of the whole system, but on the relative motion of its parts.
In front of the wire, that is, on its east side, it will be seen that as the wire approaches each portion of the medium, that portion is more and more compressed in the direction from east to west, and extended in the direction from north to south; and since the axes of the vortices lie in the north and south direction, their velocity will continually tend to increase by Prop. X., unless prevented or checked by electromotive forces acting on the circumference of each vortex.
We shall consider an electromotive force as positive when the vortices tend to move the interjacent particles upwards perpendicularly to the plane of the paper.
The vortices appear to revolve as the hands of a watch when we look at them from south to north; so that each vortex moves upwards on its west side, and downwards on its east side. In front of the wire, therefore, where each vortex is striving to increase its velocity, the electromotive force upwards must be greater on its west than on its east side. There will therefore be a continual increase of upward electromotive force from the remote east, where it is zero, to the front of the moving wire, where the upward force will be strongest.
Behind the wire a different action takes place. As the wire moves away from each successive portion of the medium, that portion is extended from east to west, and compressed from north to south, so as to tend to diminish the velocity of the vortices, and therefore to make the upward electromotive force greater on the east than on the west side of each vortex. The upward electromotive force will therefore increase continually from the remote west, where it is zero, to the back of the moving wire, where it will be strongest.
It appears, therefore, that a vertical wire moving eastwards will experience an electromotive force tending to produce in it an upward current. If there is no conducting circuit in connexion with the ends of the wire, no current will be formed, and the magnetic forces will not be altered; but if such a circuit exists, there will be a current, and the lines of magnetic force and the velocity of the vortices will be altered from their state previous to the motion of the wire. The change in the lines of force is shown in fig. 5. The vortices in front of the wire, instead of merely producing pressures, actually increase in velocity, while those behind have their velocity diminished, and those at the sides of the wire have the direction of their axes altered; so that the final effect is to produce a force acting on the wire as a resistance to its motion. We may now recapitulate the assumptions we have made, and the results we have obtained.
(1) Magneto-electric phenomena are due to the existence of matter under certain conditions of motion or of pressure in every part of the magnetic field, and not to direct action at a distance between the magnets or currents. The substance producing these effects may be a certain part of ordinary matter, or it may be an æther associated with matter. Its density is greatest in iron, and least in diamagnetic substances; but it must be in all cases, except that of iron, very rare, since no other substance has a large ratio of magnetic capacity to what we call a vacuum.
(2) The condition of any part of the field, through which lines of magnetic force pass, is one of unequal pressure in different directions, the direction of the lines of force being that of least pressure, so that the lines of force may be considered lines of tension.
(3) This inequality of pressure is produced by the existence in the medium of vortices or eddies, having their axes in the direction of the lines of force, and having their direction of rotation determined by that of the lines of force.
We have supposed that the direction was that of a watch to a spectator looking from south to north. We might with equal propriety have chosen the reverse direction, as far as known facts are concerned, by supposing resinous electricity instead of vitreous to be positive. The effect of these vortices depends on their density, and on their velocity at the circumference, and is independent of their diameter. The density must be proportional to the capacity of the substance for magnetic induction, that of the vortices in air being 1. The velocity must be very great, in order to produce so powerful effects in so rare a medium.
The size of the vortices is indeterminate, but is probably very small as compared with that of a complete molecule of ordinary matter[7].
(4) The vortices are separated from each other by a single layer of round particles, so that a system of cells is formed, the partitions being these layers of particles, and the substance of each cell being capable of rotating as a vortex.
(5) The particles forming the layer are in rolling contact with both the vortices which they separate, but do not rub against each other. They are perfectly free to roll between the vortices and so to change their place, provided they keep within one complete molecule of the substance; but in passing from one molecule to another they experience resistance, and generate irregular motions, which constitute heat. These particles, in our theory, play the part of electricity. Their motion of translation constitutes an electric current, their rotation serves to transmit the motion of the vortices from one part of the field to another, and the tangential pressures thus called into play constitute electromotive force. The conception of a particle having its motion connected with that of a vortex by perfect rolling contact may appear somewhat awkward. I do not bring it forward as a mode of connexion existing in nature, or even as that which I would willingly assent to as an electrical hypothesis. It is, however, a mode of connexion which is mechanically conceivable, and easily investigated, and it serves to bring out the actual mechanical connexions between the known electro-magnetic phenomena; so that I venture to say that any one who understands the provisional and temporary character of this hypothesis, will find himself rather helped than hindered by it in his search after the true interpretation of the phenomena.
The action between the vortices and the layers of particles is in part tangential; so that if there were any slipping or differential motion between the parts in contact, there would be a loss of the energy belonging to the lines of force, and a gradual transformation of that energy into heat. Now we know that the lines of force about a magnet are maintained for an indefinite time without any expenditure of energy; so that we must conclude that wherever there is tangential action between different parts of the medium, there is no motion of slipping between those parts. We must therefore conceive that the vortices and particles roll together without slipping; and that the interior strata of each vortex receive their proper velocities from the exterior stratum without slipping, that is, the angular velocity must be the same throughout each vortex.
The only process in which electro-magnetic energy is lost and transformed into heat, is in the passage of electricity from one molecule to another. In all other cases the energy of the vortices can only be diminished when an equivalent quantity of mechanical work is done by magnetic action.
(6) The effect of an electric current upon the surrounding medium is to make the vortices in contact with the current revolve so that the parts next to the current move in the same direction as the current. The parts furthest from the current will move in the opposite direction; and if the medium is a conductor of electricity, so that the particles are free to move in any direction, the particles touching the outside of these vortices will be moved in a direction contrary to that of the current, so that there will be an induced current in the opposite direction to the primary one.
If there were no resistance to the motion of the particles, the induced current would be equal and opposite to the primary one, and would continue as long as the primary current lasted, so that it would prevent all action of the primary current at a distance. If there is a resistance to the induced current, its particles act upon the vortices beyond them, and transmit the motion of rotation to them, till at last all the vortices in the medium are set in motion with such velocities of rotation that the particles between them have no motion except that of rotation, and do not produce currents.
In the transmission of the motion from one vortex to another, there arises a force between the particles and the vortices, by which the particles are pressed in one direction and the vortices in the opposite direction. We call the force acting on the particles the electromotive force. The reaction on the vortices is equal and opposite, so that the electromotive force cannot move any part of the medium as a whole, it can only produce currents. When the primary current is stopped, the electromotive forces all act in the opposite direction.
(7) When an electric current or a magnet is moved in presence of a conductor, the velocity of rotation of the vortices in any part of the field is altered by that motion. The force by which the proper amount of rotation is transmitted to each vortex, constitutes in this case also an electromotive force, and, if permitted, will produce currents.
(8) When a conductor is moved in a field of magnetic force, the vortices in it and in its neighbourhood are moved out of their places, and are changed in form. The force arising from these changes constitutes the electromotive force on a moving conductor, and is found by calculation to correspond with that determined by experiment.
We have now shown in what way electro-magnetic phenomena may be imitated by an imaginary system of molecular vortices. Those who have been already inclined to adopt an hypothesis of this kind, will find here the conditions which must be fulfilled in order to give it mathematical coherence, and a comparison, so far satisfactory, between its necessary results and known facts. Those who look in a different direction for the explanation of the facts, may be able to compare this theory with that of the existence of currents flowing freely through bodies, and with that which supposes electricity to act at a distance with a force depending on its velocity, and therefore not subject to the law of conservation of energy.
The facts of electro-magnetism are so complicated and various, that the explanation of any number of them by several different hypotheses must be interesting, not only to physicists, but to all who desire to understand how much evidence the explanation of phenomena lends to the credibility of a theory, or how far we ought to regard a coincidence in the mathematical expression of two sets of phenomena as an indication that these phenomena arc of the same kind. We know that partial coincidences of this kind have been discovered; and the fact that they are only partial is proved by the divergence of the laws of the two sets of phenomena in other respects. We may chance to find, in the higher parts of physics, instances of more complete coincidence, which may require much investigation to detect their ultimate divergence.
Note. — Since the first part of this paper was written, I have seen in Crelle's Journal for 1859, a paper by Prof. Helmholtz on Fluid Motion, in which he has pointed out that the lines of fluid motion are arranged according to the same laws as the lines of magnetic force, the path of an electric current corresponding to a line of axes of those particles of the fluid which are in a state of rotation. This is an additional instance of a physical analogy, the investigation of which may illustrate both electro-magnetism and hydrodynamics.
Part III: The Theory of Molecular Vortices applied to Statical Electricity
[edit]
IN the first part of this paper[8] I have shown how the forces acting between magnets, electric currents, and matter capable of magnetic induction may be accounted for on the hypothesis of the magnetic field being occupied with innumerable vortices of revolving matter, their axes coinciding with the direction of the magnetic force at every point of the field.
The centrifugal force of these vortices produces pressures distributed in such a way that the final effect is a force identical in direction and magnitude with that which we observe.
In the second part[9] I described the mechanism by which these rotations may be made to coexist, and to be distributed according to the known laws of magnetic lines of force.
I conceived the rotating matter to be the substance of certain cells, divided from each other by cell-walls composed of particles which are very small compared with the cells, and that it is by the motions of these particles, and their tangential action on the substance in the cells, that the rotation is communicated from one cell to another.
I have not attempted to explain this tangential action, but it is necessary to suppose, in order to account for the transmission of rotation from the exterior to the interior parts of each cell, that the substance in the cells possesses elasticity of figure, similar in kind, though different in degree, to that observed in solid bodies. The undulatory theory of light requires us to admit this kind of elasticity in the luminiferous medium, in order to account for transverse vibrations. We need not then be surprised if the magneto-electric medium possesses the same property.
According to our theory, the particles which form the partitions between the cells constitute the matter of electricity. The motion of these particles constitutes an electric current; the tangential force with which the particles are pressed by the matter of the cells is electromotive force, and the pressure of the particles on each other corresponds to the tension or potential of the electricity.
If we can now explain the condition of a body with respect to the surrounding medium when it is said to be "charged" with electricity, and account for the forces acting between electrified bodies, we shall have established a connexion between all the principal phenomena of electrical science.
We know by experiment that electric tension is the same thing, whether observed in statical or in current electricity; so that an electromotive force produced by magnetism may be made to charge a Leyden jar, as is done by the coil machine.
When a difference of tension exists in different parts of any body, the electricity passes, or tends to pass, from places of greater to places of smaller tension. If the body is a conductor, an actual passage of electricity takes place; and if the difference of tensions is kept up, the current continues to flow with a velocity proportional inversely to the resistance, or directly to the conductivity of the body.
The electric resistance has a very wide range of values, that of the metals being the smallest, and that of glass being so great that a charge of electricity has been preserved[10] in a glass vessel for years without penetrating the thickness of the glass.
Bodies which do not permit a current of electricity to flow through them are called insulators. But though electricity does not flow through them, electrical effects are propagated through them, and the amount of these effects differs according to the nature of the body; so that equally good insulators may act differently as dielectrics[11].
Here then we have two independent qualities of bodies, one by which they allow of the passage of electricity through them, and the other by which they allow of electrical action being transmitted through them without any electricity being allowed to pass. A conducting body may be compared to a porous membrane which opposes more or less resistance to the passage of a fluid, while a dielectric is like an elastic membrane which may be impervious to the fluid, but transmits the pressure of the fluid on one side to that on the other.
As long as electromotive force acts on a conductor, it produces a current which, as it meets with resistance, occasions a continual transformation of electrical energy into heat, which is incapable of being restored again as electrical energy by any reversion of the process.
Electromotive force acting on a dielectric produces a state of polarization of its parts similar in distribution to the polarity of the particles of iron under the influence of a magnet[12] and, like the magnetic polarization, capable of being described as a state in which every particle has its poles in opposite conditions.
In a dielectric under induction, we may conceive that the electricity in each molecule is so displaced that one side is rendered positively, and the other negatively electrical, but that the electricity remains entirely connected with the molecule, and does not pass from one molecule to another.
The effect of this action on the whole dielectric mass is to produce a general displacement of the electricity in a certain direction. This displacement does not amount to a current, because when it has attained a certain value it remains constant, but it is the commencement of a current, and its variations constitute currents in the positive or negative direction, according as the displacement is increasing or diminishing. The amount of the displacement depends on the nature of the body, and on the electromotive force; so that if is the displacement, the electromotive force, and a coefficient depending on the nature of the dielectric,
and if is the value of the electric current due to displacement,
According to our hypothesis, the magnetic medium is divided into cells, separated by partitions formed of a stratum of particles which play the part of electricity. When the electric particles are urged in any direction, they will, by their tangential action on the elastic substance of the cells, distort each cell, and call into play an equal and opposite force arising from the elasticity of the cells. When the force is removed, the cells will recover their form, and the electricity will return to its former position.
In the following investigation I have considered the relation between the displacement and the force producing it, on the supposition that the cells are spherical. The actual form of the cells probably does not differ from that of a sphere sufficiently to make much difference in the numerical result.
I have deduced from this result the relation between the statical and dynamical measures of electricity, and have shown, by a comparison of the electro-magnetic experiments of MM. Kohlrausch and Weber with the velocity of light as found by M. Fizeau, that the elasticity of the magnetic medium in air is the same as that of the luminiferous medium, if these two coexistent, coextensive, and equally elastic media are not rather one medium.
It appears also from Prop. XV. that the attraction between two electrified bodies depends on the value of , and that there- fore it would be less in turpentine than in air, if the quantity of electricity in each body remains the same. If, however, the potentials of the two bodies were given, the attraction between them would vary inversely as , and would be greater in turpentine than in air.
Prop. XII. — To find the conditions of equilibrium of an elastic sphere whose surface is exposed to normal and tangential forces, the tangential forces being proportional to the sine of the distance from a given point on the sphere.
Let the axis of z be the axis of spherical coordinates.
Let be the displacements of any particle of the sphere in the directions of x, y, and .
Let be the stresses normal to planes perpendicular to the three axes, and let be the stresses of distortion in the planes yz, zx, and .
Let be the coefficient of cubic elasticity, so that if
| (80) |
Let be the coefficient of rigidity, so that
| (81) |
Then we have the following equations of elasticity in an isotropic medium,
| (82) |
with similar equations in and , and also
| (83) |
In the case of the sphere, let us assume the radius = , and
| (84) |
Then
| (85) |
The equation of internal equilibrium with respect to is
| (86) |
which is satisfied in this case if
| (87) |
The tangential stress on the surface of the sphere, whose radius is a at an angular distance from the axis in plane ,
| (88) |
| (89) |
In order that T may be proportional to , the first term must vanish, and therefore
| (90) |
| (91) |
| (92) |
or by (87) and (90),
| (93) |
The tangential displacement of any point is
| (94) |
The normal displacement is
| (95) |
If we make
| (96) |
there will be no normal displacement, and the displacement will be entirely tangential, and we shall have
| (97) |
The whole work done by the superficial forces is
the summation being extended over the surface of the sphere.
The energy of elasticity in the substance of the sphere is
the summation being extended to the whole contents of the sphere.
We find, as we ought, that these quantities have the same value, namely
| (98) |
We may now suppose that the tangential action on the surface arises from a layer of particles in contact with it, the particles being acted on by their own mutual pressure, and acting on the surfaces of the two cells with which they are in contact.
We assume the axis of to be in the direction of maximum variation of the pressure among the particles, and we have to determine the relation between an electromotive force R acting on the particles in that direction, and the electric displacement which accompanies it.
Prop. XIII. — To find the relation between electromotive force and electric displacement when a uniform electromotive force R acts parallel to the axis of .
Take any element of the surface, covered with a stratum whose density is , and having its normal inclined to the axes of ; then the tangential force upon it will be
| (99) |
T beings as before, the tangential force on each side of the surface. Putting as in equation (34)[13], we find
| (100) |
The displacement of electricity due to the distortion of the sphere is
| taken over the whole surface; | (101) |
and if is the electric displacement per unit of volume, we shall have
| (102) |
or
| (103) |
so that
| (104) |
or we may write
| (105) |
provided we assume
| (106) |
Finding and from (87) and (90), we get
| (107) |
The ratio of to varies in different substances; but in a medium whose elasticity depends entirely upon forces acting between pairs of particles, this ratio is that of 6 to 5, and in this case
| (108) |
When the resistance to compression is infinitely greater than the resistance to distortion, as in a liquid rendered slightly elastic by gum or jelly,
| (109) |
The value of must lie between these limits. It is probable that the substance of our cells is of the former kind, and that we must use the first value of , which is that belonging to a hypothetically "perfect solid"[14] in which
| (110) |
so that we must use equation (108).
Prop. XIV. — To correct the equations (9)[15] of electric currents for the effect due to the elasticity of the medium.
We have seen that electromotive force and electric displacement are connected by equation (105). Differentiating this equation with respect to , we find
| (111) |
showing that when the electromotive force varies, the electric displacement also varies. But a variation of displacement is equivalent to a current, and this current must be taken into account in equations (9) and added to . The three equations then become
| (112) |
where p, q, r are the electric currents in the directions of x, y, and ; are the components of magnetic intensity; and P, Q, R are the electromotive forces. Now if be the quantity of free electricity in unit of volume, then the equation of continuity will be
| (113) |
Differentiating (112) with respect to x, y, and z respectively, and substituting, we find
| (114) |
whence
| (115) |
the constant being omitted, because when there are no electromotive forces.
Prop. XV.— To find the force acting between two electrified bodies.
The energy in the medium arising from the electric displacements
| (116) |
where P, Q, R are the forces, and f, g, h the displacements. Now when there is no motion of the bodies or alteration of forces, it appears from equations (77)[16] that
| (118) |
and we know by (105) that
| (119) |
whence
| (120) |
Integrating by parts throughout all space, and remembering that vanishes at an infinite distance,
| (121) |
or by (115),
| (122) |
Now let there be two electrified bodies, and let be the distribution of electricity in the first, and the electric tension due to it, and let
| (123) |
Let be the distribution of electricity in the second body, and the tension due to it; then the whole tension at any point will be , and the expansion for U will become
| (124) |
Let the body whose electricity is be moved in any way, the electricity moving along with the body, then since the distribution of tension moves with the body, the value of remains the same.
also remains the same; and Green has shown (Essay on Electricity, p. 10) that , so that the work done by moving the body against electric forces
| (125) |
And if is confined to a small body,
where F is the resistance and the motion.
If the body be small, then if is the distance from , equation (123) gives
whence
| (127) |
or the force is a repulsion varying inversely as the square of the distance.
Now let and be the same quantities of electricity measured statically, then we know by definition of electrical quantity
| (128) |
and this will be satisfied provided
| (129) |
so that the quantity E previously determined in Prop. XIII. is the number by which the electrodynamic measure of any quantity of electricity must be multiplied to obtain its electrostatic measure.
That electric current which, circulating round a ring whose area is unity, produces the same effect on a distant magnet as a magnet would produce whose strength is unity and length unity placed perpendicularly to the plane of the ring, is a unit current; and E units of electricity, measured statically, traverse the section of this current in one second, — these units being such that any two of them, placed at unit of distance, repel each other with unit of force.
We may suppose either that E units of positive electricity move in the positive direction through the wire, or that E units of negative electricity move in the negative direction, or, thirdly, that units of positive electricity move in the positive direction, while units of negative electricity move in the negative direction at the same time.
The last is the supposition on which MM. Weber and Kohlrausch[17] proceed, who have found
| (130) |
the unit of length being the millimetre, and that of time being one second, whence
| (131) |
By the ordinary method of investigation we know that
| (132) |
where is the coefficient of transverse elasticity, and is the density. By referring to the equations of Part I., it will be seen that if is the density of the matter of the vortices, and is the "coefficient of magnetic induction,"
| (133) |
whence
| (134) |
and by (108),
| (135) |
In air or vacuum =1, and therefore
| (136) |
The velocity of light in air, as determined by M. Fizeau[18], is 70,843 lenses per second (25 leagues to a degree) which gives
| (137) |
The velocity of transverse undulations in our hypothetical medium, calculated from the electro-magnetic experiments of MM. Kohlrausch and Weber, agrees so exactly with the velocity of light calculated from the optical experiments of M. Fizeau, that we can scarcely avoid the inference that light consists in the transverse undulations of the same medium which is the cause of electric and magnetic phenomena.
Prop. XVII.— To find the electric capacity of a Leyden jar composed of any given dielectric placed between two conducting surfaces.
Let the electric tensions or potentials of the two surfaces be and . Let S be the area of each surface, and the distance between them, and let and be the quantities of electricity on each surface; then the capacity
| (138) |
Within the dielectric we have the variation of perpendicular to the surface
Beyond either surface this variation is zero.
Hence by (115) applied at the surface, the electricity on unit of area is
| (139) |
and we deduce the whole capacity of the apparatus,
| (140) |
so that the quantity of electricity required to bring the one surface to a given tension varies directly as the surface, inversely as the thickness, and inversely as the square of E.
Now the coefficient of induction of dielectrics is deduced from the capacity of induction-apparatus formed of them; so that if D is that coefficient, D varies inversely as , and is unity for air. Hence
| (141) |
where V and are the velocities of light in air and in the medium. Now if is the index of refraction, , and
| (142) |
so that the inductive power of a dielectric varies directly as the square of the index of refraction, and inversely as the magnetic inductive power.
In dense media, however, the optical, electric, and magnetic phenomena may be modified in different degrees by the particles of gross matter; and their mode of arrangement may influence these phenomena differently in different directions. The axes of optical, electric, and magnetic properties will probably coincide; but on account of the unknown and probably complicated nature of the reactions of the heavy particles on the ætherial medium, it may be impossible to discover any general numerical relations between the optical, electric, and magnetic ratios of these axes.
It seems probable, however, that the value of E, for any given axis, depends upon the velocity of light whose vibrations are parallel to that axis, or whose plane of polarization is perpendicular to that axis.
In a uniaxal crystal, the axial value of E will depend on the velocity of the extraordinary ray, and the equatorial value will depend on that of the ordinary ray.
In "positive" crystals, the axial value of E will be the least and in negative the greatest.
The value of , which varies inversely as , will, cæteris paribus, be greatest for the axial direction in positive crystals, and for the equatorial direction in negative crystals, such as Iceland spar. If a spherical portion of a crystal, radius =, be suspended in a field of electric force which would act on unit of electricity with force =I, and if and be the coefficients of dielectric induction along the two axes in the plane of rotation, then if be the inclination of the axis to the electric force, the moment tending to turn the sphere will be
| (143) |
and the axis of greatest dielectric induction () will tend to become parallel to the lines of electric force.
Part IV: The Theory of Molecular Vortices applied to the Action of Magnetism on Polarized Light
[edit]
THE connexion between the distribution of lines of magnetic force and that of electric currents may be completely expressed by saying that the work done on a unit of imaginary magnetic matter, when carried round any closed curve, is proportional to the quantity of electricity which passes through the closed curve. The mathematical form of this law may be expressed as in equations (9)[19], which I here repeat, where are the rectangular components of magnetic intensity, and p, q, r are the rectangular components of steady electric currents,
| (9) |
The same mathematical connexion is found between other sets of phenomena in physical science.
(1) If represent displacements, velocities, or forces, then p, q, r will be rotatory displacements, velocities of rotation, or moments of couples producing rotation, in the elementary portions of the mass.
(2) If represent rotatory displacements in a uniform and continuous substance, then p, q, r represent the relative linear displacement of a particle with respect to those in its immediate neighbourhood. See a paper by Prof. W. Thomson "On a Mechanical Representation of Electric, Magnetic, and Galvanic Forces," Camb. and Dublin Math. Journ. Jan. 1847.
(3) If represent the rotatory velocities of vortices whose centres are fixed, then p, q, r represent the velocities with which loose particles placed between them would be carried along. See the second part of this paper (Phil. Mag. April 1861).
It appears from all these instances that the connexion between magnetism and electricity has the same mathematical form as that between certain pairs of phenomena, of which one has a linear and the other a rotatory character. Professor Challis[20] conceives magnetism to consist in currents of a fluid whose direction corresponds with that of the lines of magnetic force; and electric currents, on this theory, are accompanied by, if not dependent on, a rotatory motion of the fluid about the axes of the current. Professor Helmholtz[21] has investigated the motion of an incompressible fluid, and has conceived lines drawn so as to correspond at every point with the instantaneous axis of rotation of the fluid there. He has pointed out that the lines of fluid motion are arranged according to the same laws with respect to the lines of rotation, as those by which the lines of magnetic force are arranged with respect to electric currents. On the other hand, in this paper I have regarded magnetism as a phenomenon of rotation, and electric currents as consisting of the actual translation of particles, thus assuming the inverse of the relation between the two sets of phenomena.
Now it seems natural to suppose that all the direct effects of any cause which is itself of a longitudinal character, must be themselves longitudinal, and that the direct effects of a rotatory cause must be themselves rotatory. A motion of translation along an axis cannot produce a rotation about that axis unless it meets with some special mechanism, like that of a screw, which connects a motion in a given direction along the axis with a rotation in a given direction round it; and a motion of rotation, though it may produce tension along the axis, cannot of itself produce a current in one direction along the axis rather than the other.
Electric currents are known to produce effects of transference in the direction of the current. They transfer the electrical state from one body to another, and they transfer the elements of electrolytes in opposite directions, but they do not[22] cause the plane of polarization of light to rotate when the light traverses le axis of the current.
On the other hand, the magnetic state is not characterized by any strictly longitudinal phenomenon. The north and south poles differ only in their names, and these names might be exchanged without altering the statement of any magnetic phenomenon; whereas the positive and negative poles of a battery are completely distinguished by the different elements of water which are evolved there. The magnetic state, however, is characterized by a well-marked rotatory phenomenon discovered by Faraday[23] — the rotation of the plane of polarized light when transmitted along the lines of magnetic force.
When a transparent diamagnetic substance has a ray of plane-polarized light passed through it, and if lines of magnetic force are then produced in the substance by the action of a magnet or of an electric current, the plane of polarization of the transmitted light is found to be changed, and to be turned through an angle depending on the intensity of the magnetizing force within we substance.
The direction of this rotation in diamagnetic substances is the same as that in which positive electricity must circulate round the substance in order to produce the actual magnetizing force within it; or if we suppose the horizontal part of terrestrial magnetism to be the magnetizing force acting on the substance, the plane of polarization would be turned in the direction of the earth's true rotation, that is, from west upwards to east.
In paramagnetic substances, M. Verdet[24] has found that the plane of polarization is turned in the opposite direction, that is, in the direction in which negative electricity would flow if the magnetization were effected by a helix surrounding the substance.
In both cases the absolute direction of the rotation is the same, whether the light passes from north to south or from south to north,— a fact which distinguishes this phenomenon from the rotation produced by quartz, turpentine, &c., in which the absolute direction of rotation is reversed when that of the light is reversed. The rotation in the latter case, whether related to an axis, as in quartz, or not so related, as in fluids, indicates a relation between the direction of the ray and the direction of rotation, which is similar in its formal expression to that between the longitudinal and rotatory motions of a right-handed or a left-handed screw; and it indicates some property of the substance the mathematical form of which exhibits right-handed or left-handed relations, such as are known to appear in the external forms of crystals having these properties. In the magnetic rotation no such relation appears, but the direction of rotation is directly connected with that of the magnetic lines, in a way which seems to indicate that magnetism is really a phenomenon of rotation.
The transference of electrolytes in fixed directions by the electric current, and the rotation of polarized light in fixed directions by magnetic force, are the facts the consideration of which has induced me to regard magnetism as a phenomenon of rotation, and electric currents as phenomena of translation, instead of following out the analogy pointed out by Helmholtz, or adopting the theory propounded by Professor Challis.
The theory that electric currents are linear, and magnetic forces rotatory phenomena, agrees so far with that of Ampère and Weber; and the hypothesis that the magnetic rotations exist wherever magnetic force extends, that the centrifugal force of these rotations accounts for magnetic attractions, and that the inertia of the vortices accounts for induced currents, is supported by the opinion of Professor W. Thomson[25]. In fact the whole theory of molecular vortices developed in this paper has been suggested to me by observing the direction in which those investigators who study the action of media are looking for the explanation of electro-magnetic phenomena.
Professor Thomson has pointed out that the cause of the magnetic action on light must be a real rotation going on in the magnetic field. A right-handed circularly polarized ray of light is found to travel with a different velocity according as it passes from north to south, or from south to north, along a line of magnetic force. Now, whatever theory we adopt about the direction of vibrations in plane-polarized light, the geometrical arrangement of the parts of the medium during the passage of a right-handed circularly polarized ray is exactly the same whether the ray is moving north or south. The only difference is, that the particles describe their circles in opposite directions. Since, therefore, the configuration is the same in the two cases, the forces acting between particles must be the same in both, and the motions due to these forces must be equal in velocity if the medium was originally at rest; but if the medium be in a state of rotation, either as a whole or in molecular vortices, the circular vibrations of light may differ in velocity according as their direction is similar or contrary to that of the vortices.
We have now to investigate whether the hypothesis developed in this paper — that magnetic force is due to the centrifugal force of small vortices, and that these vortices consist of the same matter the vibrations of which constitute light — leads to any conclusions as to the effect of magnetism on polarized light. We suppose transverse vibrations to be transmitted through a magnetized medium. How will the propagation of these vibrations be affected by the circumstance that portions of that medium are in a state of rotation?
In the following investigation, I have found that the only effect which the rotation of the vortices will have on the light will be to make the plane of polarization rotate in the same direction as the vortices, through an angle proportional —
(A) to the thickness of the substance,
(B) to the resolved part of the magnetic force parallel to the ray,
(C) to the index of refraction of the ray,
(D) inversely to the square of the wave-length in air,
(E) to the mean radius of the vortices,
(F) to the capacity for magnetic induction.
A and B have been fully investigated by M. Verdet[26], who has shown that the rotation is strictly proportional to the thickness and to the magnetizing force, and that, when the ray is inclined to the magnetizing force, the rotation is as the cosine of that inclination. D has been supposed to give the true relation between the rotation of different rays; but it is probable that C must betaken into account in an accurate statement of the phenomena: The rotation varies, not exactly inversely as the square of the wave-length, but a little faster; so that for the highly refrangible rays the rotation is greater than that given by this law, but more nearly as the index of refraction divided by the square of the wave-length.
The relation (E) between the amount of rotation and the size of the vortices shows that different substances may differ in rotating power independently of any observable difference in other, respects. We know nothing of the absolute size of the vortices; and on our hypothesis the optical phenomena are probably the only data for determining their relative size in different substances.
On our theory, the direction of the rotation of the plane of polarization depends on that of the mean moment of momenta, or angular momentum, of the molecular vortices ; and since M. Verdet has discovered that magnetic substances have an effect on light opposite to that of diamagnetic substances, it follows that the molecular rotation must be opposite in the two classes of substances.
We can no longer, therefore, consider diamagnetic bodies as. being those whose coefficient of magnetic induction is less than that of space empty of gross matter. We must admit the diamagnetic state to be the opposite of the paramagnetic; and that the vortices, or at least the influential majority of them, in diamagnetic substances, revolve in the direction in which positive electricity revolves in the magnetizing bobbin, while in paramagnetic substances they revolve in the opposite direction.
This result agrees so far with that part of the theory of M. Weber[27] which refers to the paramagnetic and diamagnetic conditions. M. Weber supposes the electricity in paramagnetic bodies to revolve the same way as the surrounding helix, while in diamagnetic bodies it revolves the opposite way. Now if we regard negative or resinous electricity as a substance the absence of which constitute positive or vitreous electricity, the results will be those actually observed. This will be true independently of any other hypothesis than that of M. Weber about magnetism and diamagnetism, and does not require us to admit either M. Weber's theory of the mutual action of electric particles in motion or our theory of cells and cell-walls.
I am inclined to believe that iron differs from other substances in the manner of its action as well as in the intensity of its magnetism; and I think its behaviour may be explained on our hypothesis of molecular vortices, by supposing that the particles of the iron itself are set in rotation by the tangential action of the vortices, in an opposite direction to their own. These large heavy particles would thus be revolving exactly as we have supposed the infinitely small particles constituting electricity to revolve, but without being free like them to change their place and form currents.
The whole energy of rotation of the magnetised field would thus be greatly increased, as we know it to be; but the angular momentum of the iron particles would be opposite to that of the ætherial cells and immensely greater, so that the total angular momentum of the substance will be in the direction of rotation of the iron, or the reverse of that of the vortices. Since, however, the angular momentum depends on the absolute size of the revolving portions of the substance, it may depend on the state of aggregation or chemical arrangement of the elements, as well as on the ultimate nature of the components of the substance. Other phenomena in nature seem to lead to the conclusion that all substances are made up of a number of parts, finite in size, the particles composing these parts being themselves capable of internal motion.
Prop. XVIII. — To find the angular momentum of a vortex.
The angular momentum of any material system about an axis is the sum of the products of the mass, , of each particle multiplied by twice the area it describes about that axis in unit of time; or if A is the angular momentum about the axis of ,
As we do not know the distribution of density within the vortex, we shall determine the relation between the angular momentum and the energy of the vortex which was found in Prop. VI.
Since the time of revolution is the same throughout the vortex, the mean angular velocity will be uniform and , where is the velocity at the circumference, and the radius. Then
| [28] | (144) |
whence
| (144) |
for the axis of , with similar expressions for the other axes, V being the volume, and the radius of the vortex.
Prop. XIX. — To determine the conditions of undulatory motion in a medium containing vortices, the vibrations being perpendicular to the direction of propagation.
Let the waves be plane-waves propagated in the direction of , and let the axis of and be taken in the directions of greatest and least elasticity in the plane . Let and represent the displacement paralll to these axes, which will be the same throughout the same wave-surface, and therefore we shall have and functions of and only.

Let X be the tangential stress on unit of area parallel to , tending to move the part next the origin in the direction of .
Let Y be the corresponding tangential stress in the direction of .
Let and be the coefficients of elasticity with respect to these two kinds of tangential stress; then, if the medium is at rest,
Now let us suppose vortices in the medium whose velocities are represented as usual by the symbols , and let us suppose that the value of is increasing at the rate , on account of the action of the tangential stresses alone, there being no electromotive force in the field. The angular momentum in the stratum whose area is unity, and thickness , is therefore increasing at the rate ; and if the part of the force Y which produces this effect is Y', then the moment of Y' is ,so that .
The complete value of Y when the vortices are in a state of varied motion is
| (145) |
The whole force acting upon a stratum whose thickness is and area unity, is in the direction of , and in direction of . The mass of the stratum is , so that we have as the equations of motion,
| (146) |
Now the changes of velocity and are produced by the motion of the medium containing the vortices, which distorts and twists every element of its mass; so that we must refer to Prop. X.[29] to determine these quantities in terms of the motion. We find there at equation (68),
| (68) |
Since and are functions of and only, we may write this equation
| (147) |
so that if we now put , and , we may write the equations of motion
| (148) |
These equations may be satisfied by the values provided
| (149) |
| (150) |
Multiplying the last two equations together, we find
| (151) |
an equation quadratic with respect to , the solution of which is
| (152) |
These values of being put in the equations (150) will each give a ratio of A and B,
which being substituted in equations (149), will satisfy the original equations (148). The most general undulation of such a medium is therefore compounded of two elliptic undulations of different eccentricities travelling with different velocities and rotating in opposite directions. The results may be more easily explained in the case in which ; then
| (153) |
Let us suppose that the value of A is unity for both vibrations, then we shall have
| (154) |
The first terms of and represent a circular vibration in the negative direction, and the second term a circular vibration in the positive direction, the positive having the greatest velocity of propagation. Combining the terms, we may write
| (155) |
where
| (156) |
These are the equations of an undulation consisting of a plane vibration whose periodic time is , and wave-length , propagated in the direction of with a velocity , while the plane of the vibration revolves about the axis of in the positive direction so as to complete a revolution when .
Now let us suppose small, then we may write
| (157) |
and remembering that , we find
| (158) |
Here is the radius of the vortices, an unknown quantity. is the density of the luminiferous medium in the body, which is also unknown; but if we adopt the theory of Fresnel, and make the density in space devoid of gross matter, then
| (159) |
where is the index of refraction.
On the theory of MacCullagh and Neumann,
| (160) |
in all bodies.
is the coefficient of magnetic induction, which is unity in empty space or in air.
is the velocity of the vortices at their circumference estimated in the ordinary units. Its value is unknown, but it is proportional to the intensity of the magnetic force.
Let Z be the magnetic intensity of the field, measured as in the case of terrestrial magnetism, then the intrinsic energy in air per unit of volume is
where is the density of the magnetic medium in air, which we have reason to believe the same as that of the luminiferous medium. We therefore put
| (161) |
is the wave-length of the undulation in the substance. Now if be the wavelength for the same ray in air, and the index of refraction of that ray in the body,
| (162) |
Also , the velocity of light in the substance, is related to V, the velocity of light in air, by the equation
| (163) |
Hence if be the thickness of the substance through which the ray passes, the angle through which the plane of polarization will be turned will be in degrees,
| (164) |
or, by what we have now calculated,
| (165) |
In this expression all the quantities are known by experiment except , the radius of the vortices in the body, and , the density of the luminiferous medium in air.
The experiments of M, Verdet[30] supply all that is wanted except the determination of Z in absolute measures and this would also be known for all his experiments, if the value of the galvanometer deflection for a semirotation of the testing bobbin in a known magnetic field, such as that due to terrestrial magnetism at Paris, were once for all determined.
Footnotes
[edit]- ↑ See a paper "On Faraday's Lines of Force," Cambridge Philosophical Transactions, vol. x. part I.
- ↑ See Faraday's 'Researches,' 3252.
- ↑ Rankine's 'Applied Mechanics,' art. 106.
- ↑ Rankine's 'Applied Mechanics,' art. 116.
- ↑ See Goodeve's 'Elements of Mechanism,' p. 118.
- ↑ Cambridge Philosophical Transactions, vol. x. part 1. art. 3, "On Faraday's Lines of Force."
- ↑ The angular momentum of the system of vortices depends on their average diameter; so that if the diameter were sensible, we might expect that a magnet would behave as if it contained a revolving body within it, and that the existence of this rotation might be detected by experiments on the free rotation of a magnet. I have made experiments to investigate this question, but have not yet fully tried the apparatus.
- ↑ Phil. Mag. March 1861.
- ↑ Phil. Mag. April and May 1861.
- ↑ By Professor W. Thomson.
- ↑ Faraday, ' Experimental Researches,' Series XI.
- ↑ See Prof. Mossotti, "Discussione Analitica," Memorie della Soc. Italiana (Modena), vol. xxiv. part 2. p. 49.
- ↑ Phil. Mag. April 1861.
- ↑ See Rankine "On Elasticity," Camb. and Dub. Math. Journ. 1851.
- ↑ Phil. Mag. March 1861.
- ↑ Phil. Mag. May 1861.
- ↑ Abhandlungen der König. Sächsischen Gesellschaft, vol. iii. (1857). p. 260.
- ↑ Comptes Rendus, vol. xxix. (1849), p. 90. In Galbraith and Haughton's 'Manual of Astronomy,' M. Fizeau's result is stated at 169,944 geographical miles of 1000 fathoms, which gives 193,118 statute miles; the value deduced from aberration is 192.000 miles.
- ↑ Phil. Mag. March 1861.
- ↑ Phil. Mag. December 1860, January and February 1861.
- ↑ Crelle, Journal, vol. lv. (1858) p. 25.
- ↑ Faraday, 'Experimental Researches,' 951-954, and 2216-2220.
- ↑ Ibid., Series XIX.
- ↑ Comptes Rendus, vol. xliii. p. 529; vol. xliv. p. 1209.
- ↑ See Nichol's Cyclopadia, art. "Magnetism, Dynamical Relations of," edition 1860; Proceedings of Royal Society, June 1856 and June 1861; and Phil. Mag. 1857.
- ↑ Annales de Chimie et de Physique, ser. 3. vol. xli. p. 370; vol. xliii. p. 37.
- ↑ Taylor's 'Scientific Memoirs,' vol. v. p. 477.
- ↑ Phil. Mag. April 1861.
- ↑ Phil. Mag. May 1861.
- ↑ Annales de Chimie et de Physique, ser. 3, vol. xli p. 370.
![]()
This work was published before January 1, 1929, and is in the public domain worldwide because the author died at least 100 years ago.
Public domainPublic domainfalsefalse



































































































































































































































































































































































































